+ - 0:00:00
Notes for current slide
Notes for next slide

2.4 — Exogenous Growth Theory

ECON 317 • Economic Development • Fall 2021

Ryan Safner
Assistant Professor of Economics
safner@hood.edu
ryansafner/devF21
devF21.classes.ryansafner.com

Outline

Neoclassical Working Tools

The “Simple” Solow Model

The “Full” Solow Model

Cross-Country Comparisons

Growth Accounting

Neoclassical Working Tools

An Aggregate Production Function I

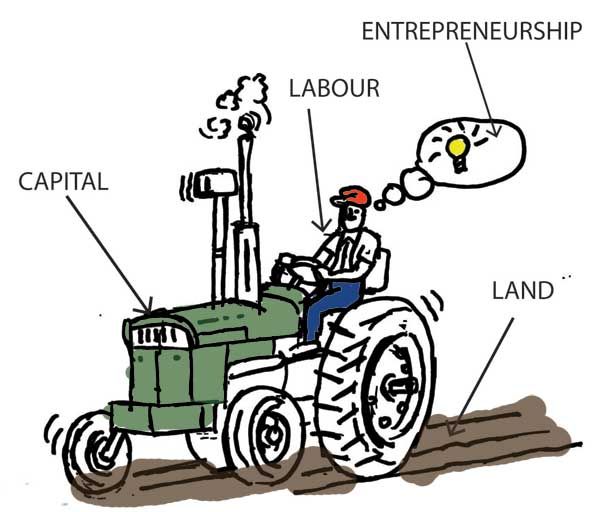
  • Aggregate production function: rate at which an economy converts inputs into output1

Y=A∗f(L,K,T)

  • Economists often categorize inputs:
Factor Owned By Earns
Land (T) Landowners Rent
Labor (L) Laborers Wages
Capital (K) Capitalists Interest
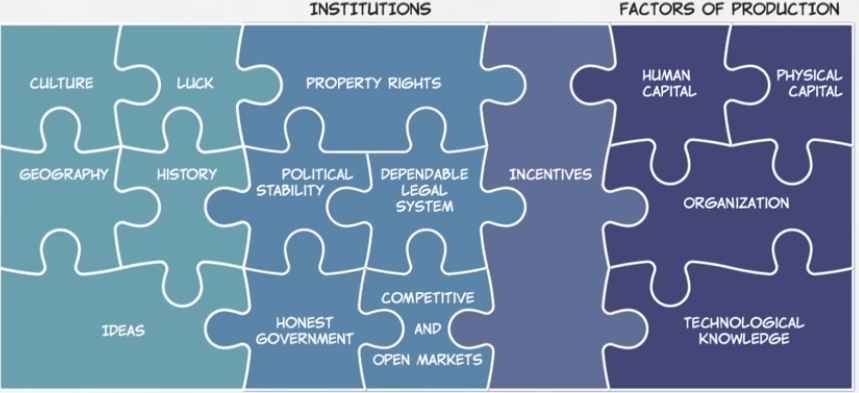
  • “A” is called total factor productivity, augments all factors to improve output
    • Often called “technology” but more like “ideas, incentives, & institutions”

Theoretical Microfoundations I

  • Assume N firms (i=1,2,⋯,N) all have the same production technology yi=a∗f(Li,Ki,Ti)

  • All firms minimize cost of production and face the same factor prices:1

    • pL=w=MPL
    • pK=i=MPK
    • pT=r=MPT

1 Assuming competitive markets, all factor prices (wages, interest, rents) are equal to the marginal productivity of labor, capital, and land, respectively.

Theoretical Microfoundations II

  • The economy behaves “as if” there is a single firm with technology: Y=A∗f(L,K,T)
    and facing factor prices, where aggregate inputs and output are:

L=l1+l2+⋯+lNK=k1+k2+⋯+kNT=t1+t2+⋯+tNY=y1+y2+⋯+yN

An Aggregate Production Function: Implications

Assuming constant returns to scale (output and all inputs scale at the same proportionate rate):

  • If two countries have the same technology, there is no economic advantage to size

  • Labor productivity (YL), output-per-worker/hour, is determined only by (KL), capital-per-worker/hour

Y=MPLL+MPKK+MPTT

  • With competitive markets, firms pay each factor its marginal product, firms earn no profits1

1 This is also called the "product exhaustion theorem," and comes from Euler's Theorem for homogeneous functions (constant returns functions are homogeneous of degree 1).

An Aggregate Production Function: Cobb-Douglas I

  • Common functional form in economics: Cobb-Douglas

Y=AKαL1−α

  • Exponents (α) and (1−α) are “output-elasticities”

    • A 1% increase in K (L) will yield an α% (1−α) increase in Y

  • Constant returns to scale1: a k% increase in all inputs will yield a k% increase in Y

  • More about Cobb-Douglas functions

Y=K0.5L0.5

1 Only when all exponents sum to 1. In technical terms, the production function is "homogeneous of degree 1"

An Aggregate Production Function: Cobb-Douglas I

Y=AKαL1−α

  • GDP (Y): “Total Output” = “Total Income” for all factor-owners

  • Exponents α and (1−α) are the Factor Shares of National Income

    • α: capital's share of national income
    • (1−α): labor's share of national income

  • Empirically, very stable:

    • Capital's share: α≈0.3
    • Labor's share: 1−α≈0.7

Y=K0.3L0.7

Aggregate Production Function: Labor I

  • Look at Labor, holding other factors constant:1

Example: When ˉK=9

Y=3L0.5

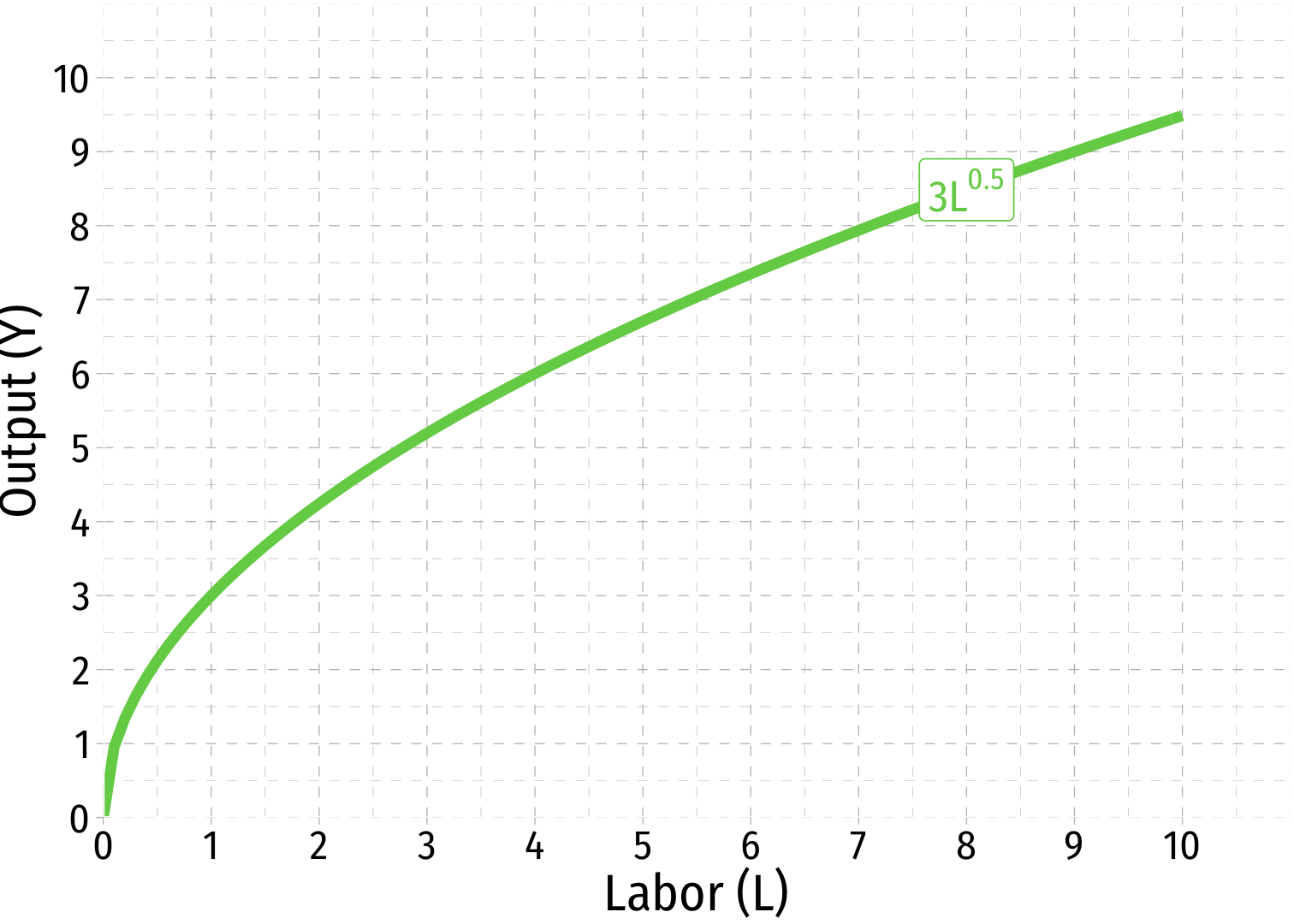
1 We often consider "the short run" where K is fixed, and production functions are simply functions of labor with fixed capital y=f(ˉk,l).

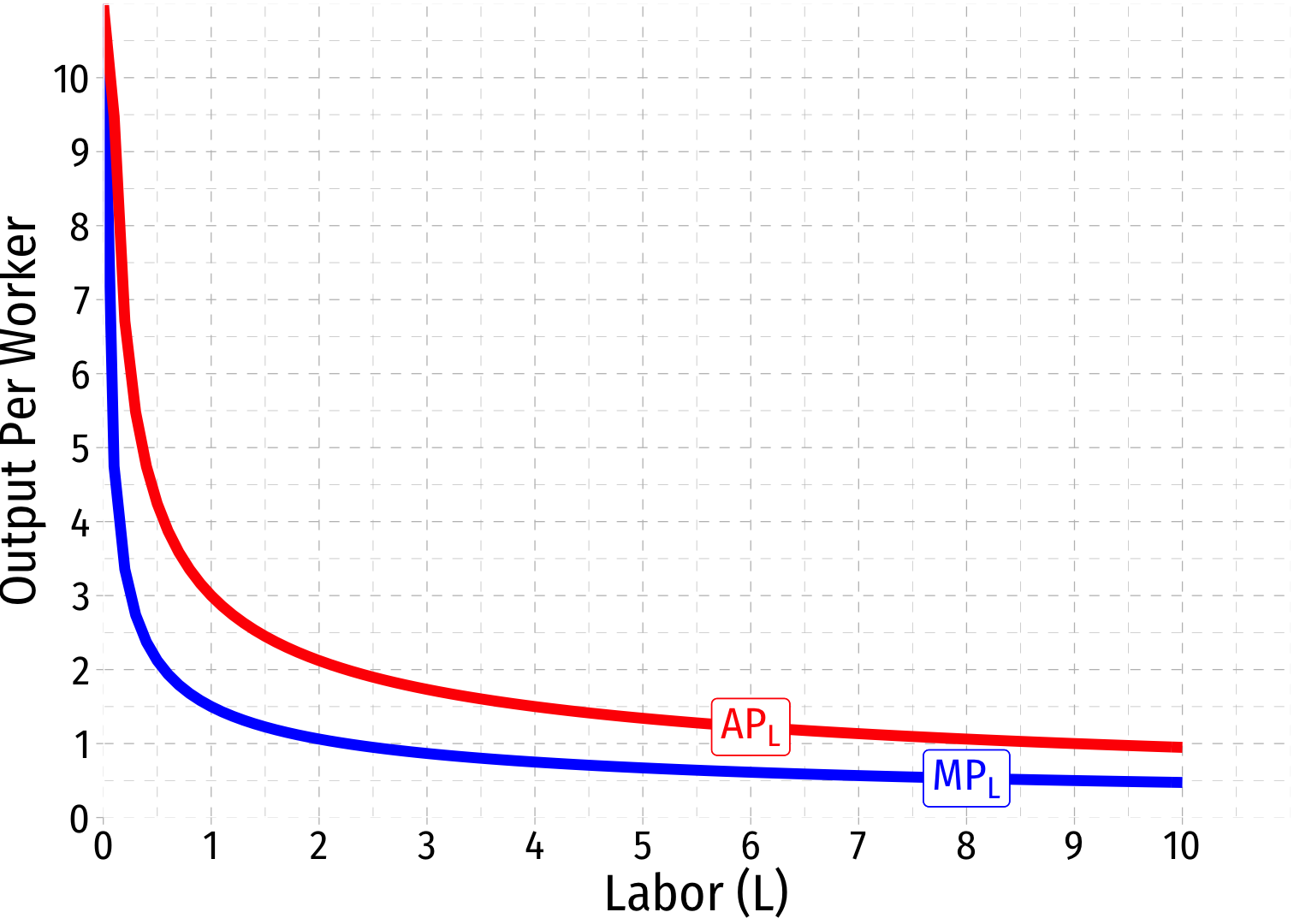
Aggregate Production Function: Labor II

  • Look at Labor, holding other factors constant:

  • The marginal product of labor: the additional output produced by an additional unit of labor (holding other factors constant)

MPL=ΔYΔL

  • The average product of labor: output per worker

APL=YL

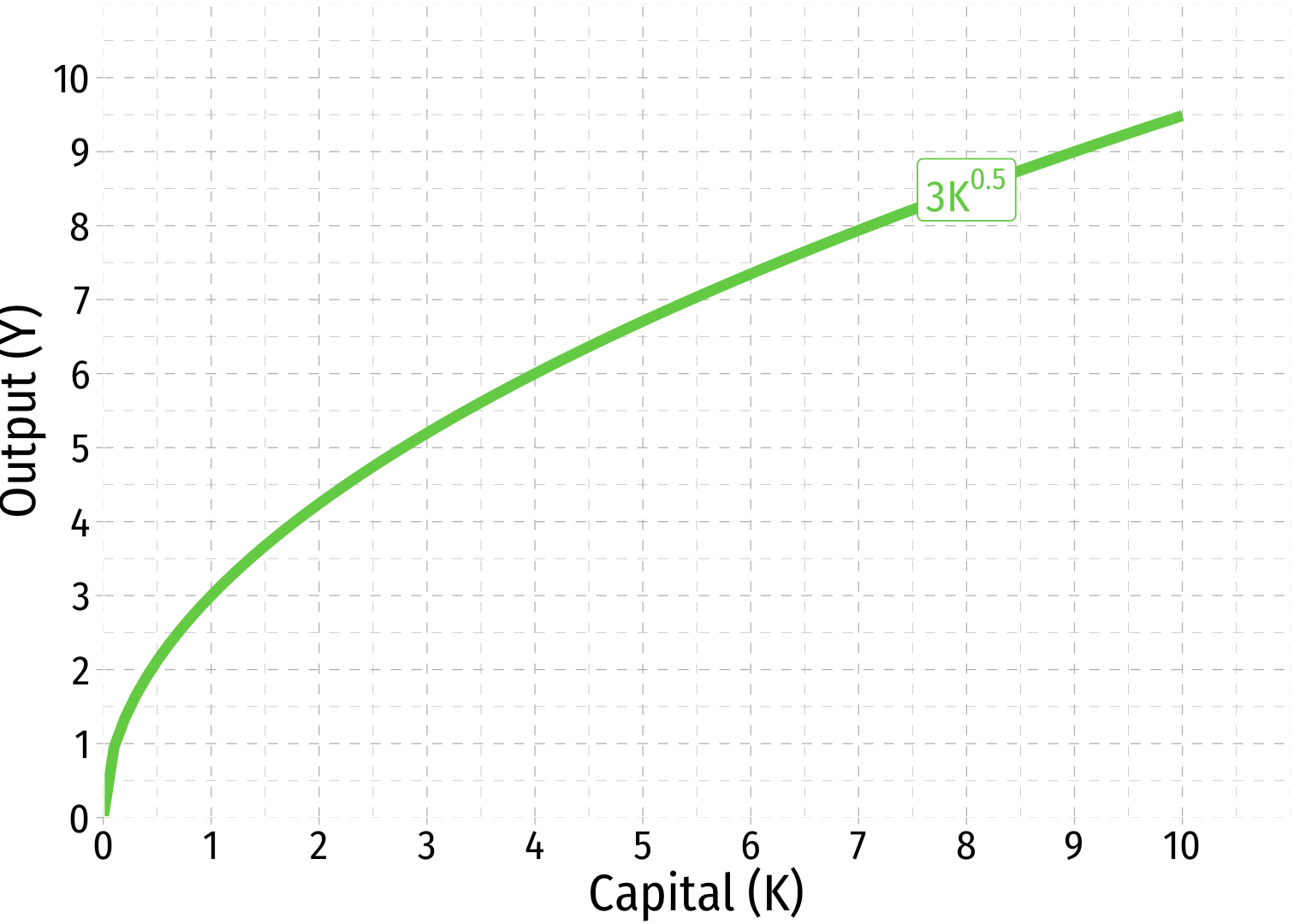
Aggregate Production Function: Capital I

  • Look at Capital, holding other factors constant:

Example: When ˉL=9

Y=3K0.5

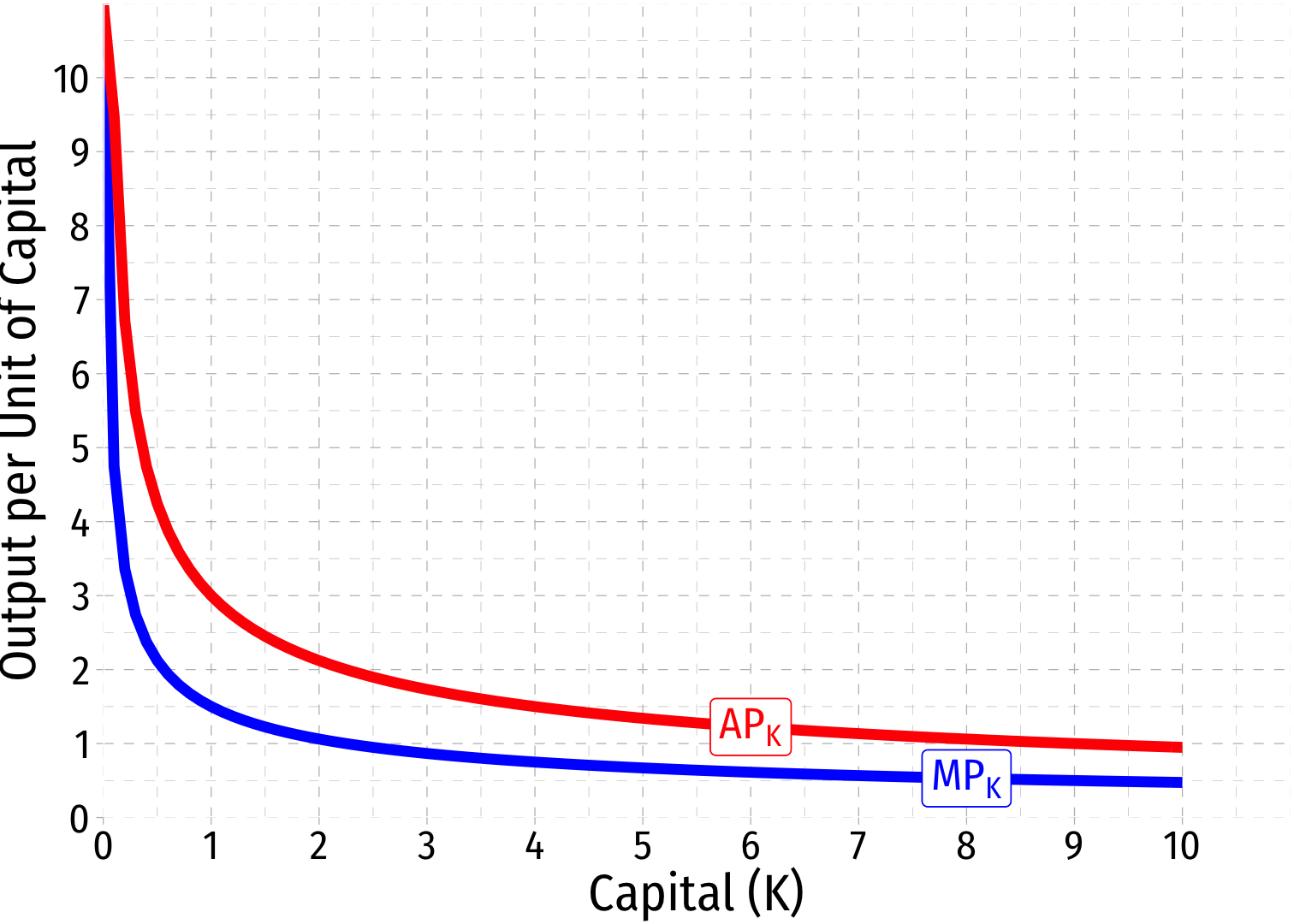
Aggregate Production Function: Capital II

  • The marginal product of capital: the additional output produced by an additional unit of capital (holding other factors constant)

MPK=ΔYΔK

  • The average product of capital: output per unit of capital

APK=YK

Capital and Labor

  • Often compare capital-to-labor ratio (KL)

  • Capital "widening": stock of capital increases, but capital per worker (KL) does not change

    • Increase in K is same rate as increase in labor and depreciation

  • Capital "deepening": stock of capital per worker (KL) is increasing

The Solow Model

Kaldor's Stylized Facts About Growth

Nicholas Kaldor

(1908-1986)

"A satisfactory model concerning the nature of the growth process in a capitalist economy must also account for the remarkable historical constancies revealed by recent empirical investigations." (p.591)

  1. Output per worker grows over time

  2. Capital per worker grows over time

  3. The capital-to-output ratio is approximately constant over time

  4. Capital and labor's share of output is approximately constant over time

  5. The return to capital is approximately constant over time

  6. Levels of output per person vary widely across countries

Kaldor, Nicholas, 1957, "A Model of Economic Growth," Economic Journal 67(268): 591-624

The Solow (Neoclassical) Growth Model

Robert Solow

(1924-)

Economics Nobel 1987

"All theory depends on assumptions which are not quite true. That is what makes it theory. The art of successful theorizing is to make the inevitable simplifying assumptions in such a way that the final results are not very sensitive," (p.65)

"The characteristic and powerful conclusion of the Harrod-Domar line of thought is that even for the long run the economic system is at best balanced on a knife-edge of equilibrium growth...The bulk of [Solow's] paper is devoted to a model of long-run growth which accepts all of the Harrod-Domar assumptions [but] instead I suppose that [output] is produced by labor and capital under the standard neoclassical conditions," (pp.65-66)

Solow, Robert, 1956, "A Contribution to the Theory of Economic Growth," Quarterly Journal of Economics 70(1): 65-94

The “Simple” Solow Model: Key Assumptions

  • An aggregate Cobb-Douglas production function
  • Diminishing returns to factors, ↓MPL and ↓MPK
  • Can accumulate physical capital (K)
  • Technology grows exogenously (some fixed rate determined outside of the model)

  • Constant rate of Savings and of Investment (s)

  • I am going to leave out excess parts of the model: role of taxes, interest rates, etc, on consumption, saving, and investment1

1 This isn't a macroeconomics course!

The “Simple” Solow Model: Equations

(1) Ct+It=Yt=f(K,L)

  • Income is equal to consumption plus investment
  • Output is equal to the production function
  • Income = Output

The “Simple” Solow Model: Equations

(1) Ct+It=Yt=f(K,L)

  • Income is equal to consumption plus investment
  • Output is equal to the production function
  • Income = Output

(2) It=sf(Kt,Lt)

  • Investment is equal to the fraction of income (output) saved s times output

The “Simple” Solow Model: Equations

(1) Ct+It=Yt=f(K,L)

  • Income is equal to consumption plus investment
  • Output is equal to the production function
  • Income = Output

(2) It=sf(Kt,Lt)

  • Investment is equal to the fraction of income (output) saved s times output

(3) Kt+1=Kt(1−δ)+It

  • The stock of capital K changes over time from depreciation (δ) and new investment It

The “Simple” Solow Model: Equations

(1) Ct+It=Yt=f(K,L)

  • Income is equal to consumption plus investment
  • Output is equal to the production function
  • Income = Output

(2) It=sf(Kt,Lt)

  • Investment is equal to the fraction of income (output) saved s times output

(3) Kt+1=Kt(1−δ)+It

  • The stock of capital K changes over time from depreciation (δ) and new investment It

(4) Lt=L

  • The stock of labor is constant over time

The “Simple” Solow Model: Implications

  • Capital growth over time:

Kt+1=Kt(1−δ)+sf(Kt,Lt)

  • Plugging equation 2 into equation 3

The “Simple” Solow Model: Implications

  • Capital growth over time:

Kt+1=Kt(1−δ)+sf(Kt,Lt)

  • Plugging equation 2 into equation 3
  • Steady-State equilibrium: δK=sf(K,L)
    • Amount of capital depreciation is equal to the amount saved & invested in new capital formation
    • Capital growth “breaks even” to have a constant amount of K over time

Equations and Implications, in Terms of k

  • Restate model in terms of k≡KL, i.e. divide everything by L to get “per worker”
    • y=YL, output per worker
    • k=KL, capital per worker

Equations and Implications, in Terms of k

  • Restate model in terms of k≡KL, i.e. divide everything by L to get “per worker”
    • y=YL, output per worker
    • k=KL, capital per worker

  1. ct+it=yt=f(kt)

  2. it=sf(kt)

  3. kt+1=kt(1−δ)+it

Equations and Implications, in Terms of k

  • Restate model in terms of k≡KL, i.e. divide everything by L to get “per worker”
    • y=YL, output per worker
    • k=KL, capital per worker

  1. ct+it=yt=f(kt)

  2. it=sf(kt)

  3. kt+1=kt(1−δ)+it

  • Implications

  1. kt+1=kt(1−δ)+sf(kt)

  2. Steady-State equilibrium: δk=sf(k)

Graphically: Capital and Depreciation I

  • Whenever Investment > Depreciation:
    • Capital stock is growing over time, gK>0
    • Adding more new capital than is lost to depreciation
    • Movement to the right on graph k→

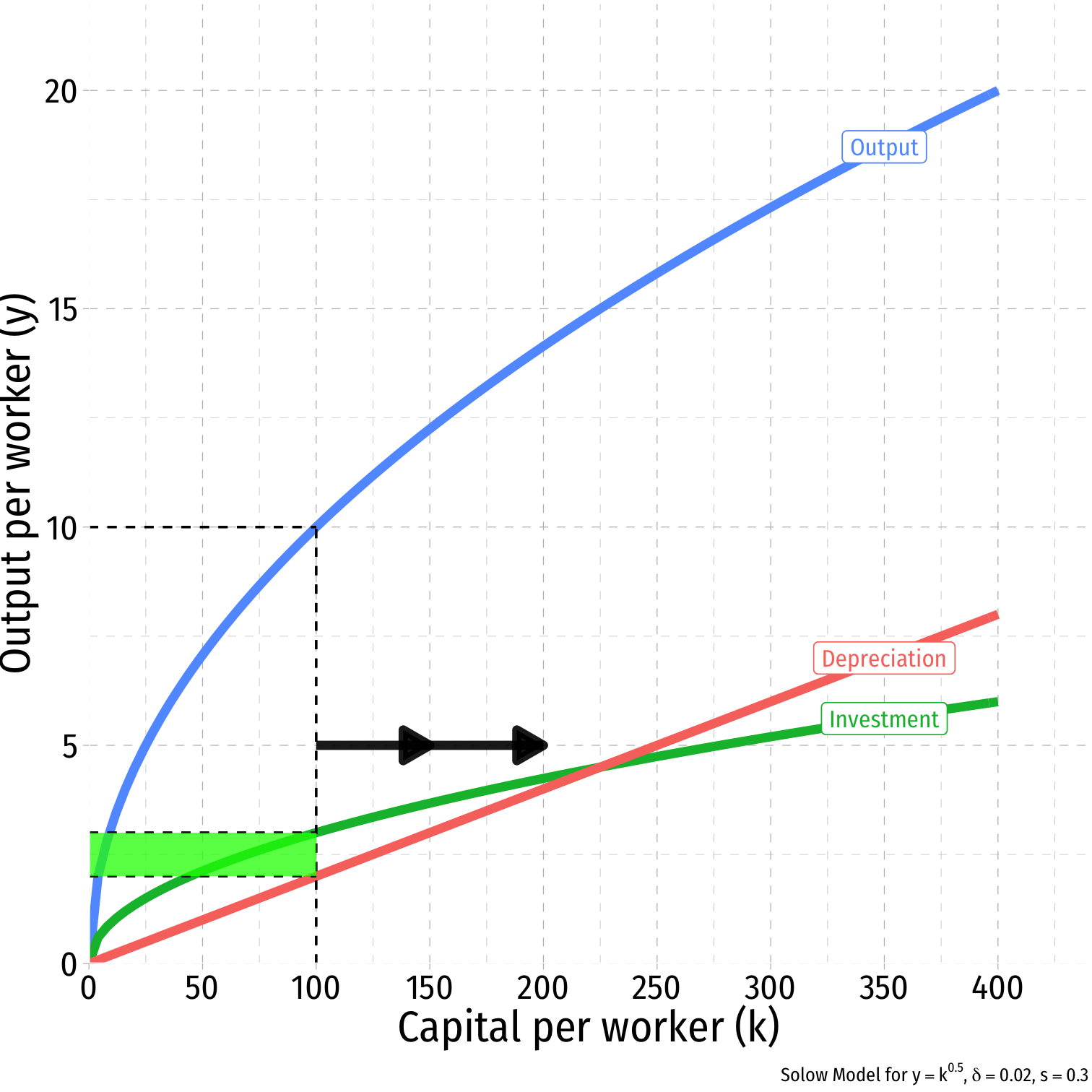
Graphically: Capital and Depreciation II

  • Whenever Investment < Depreciation:
    • Capital stock is shrinking over time, gK<0
    • Adding less new capital than is lost to depreciation
    • Movement to the left on graph ←k

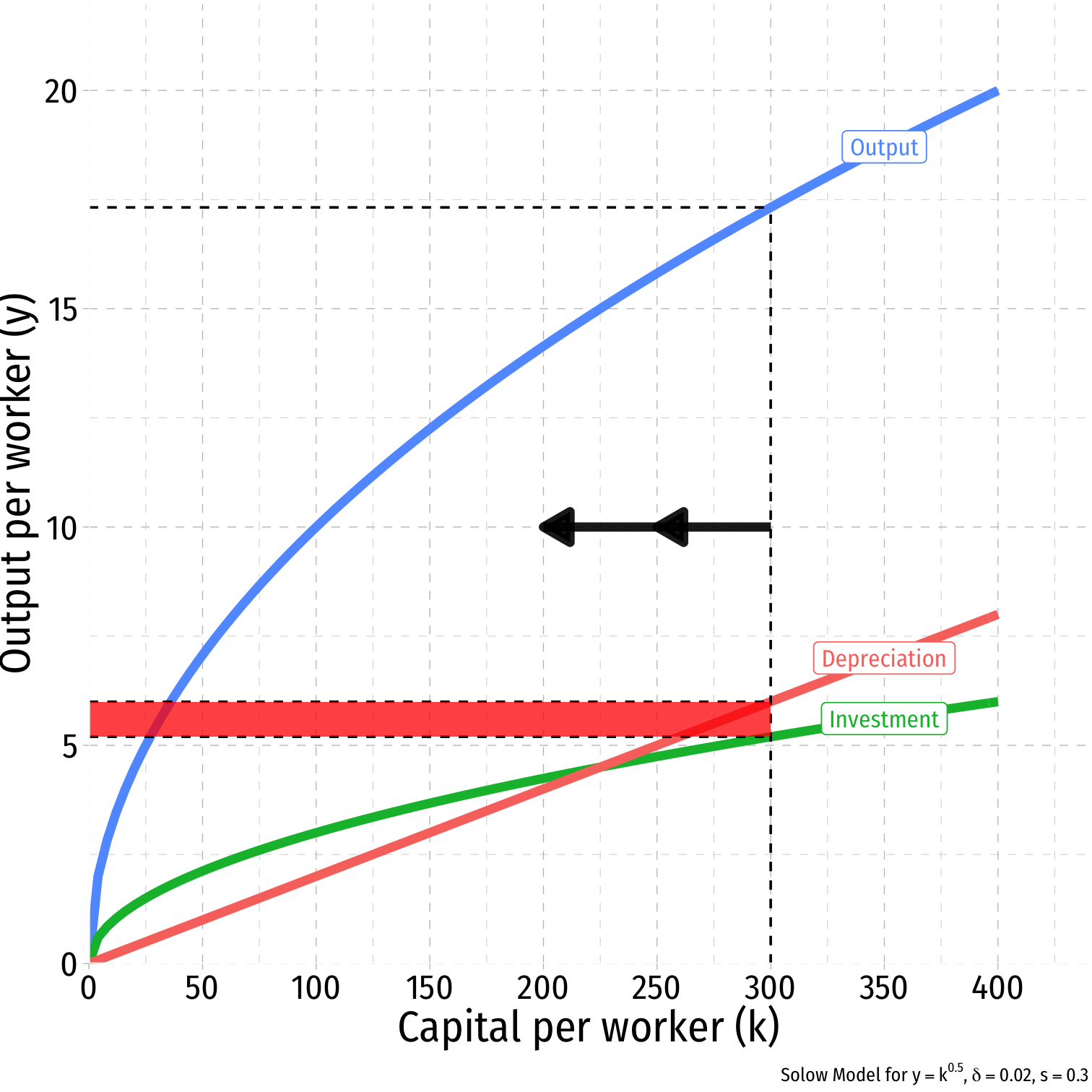
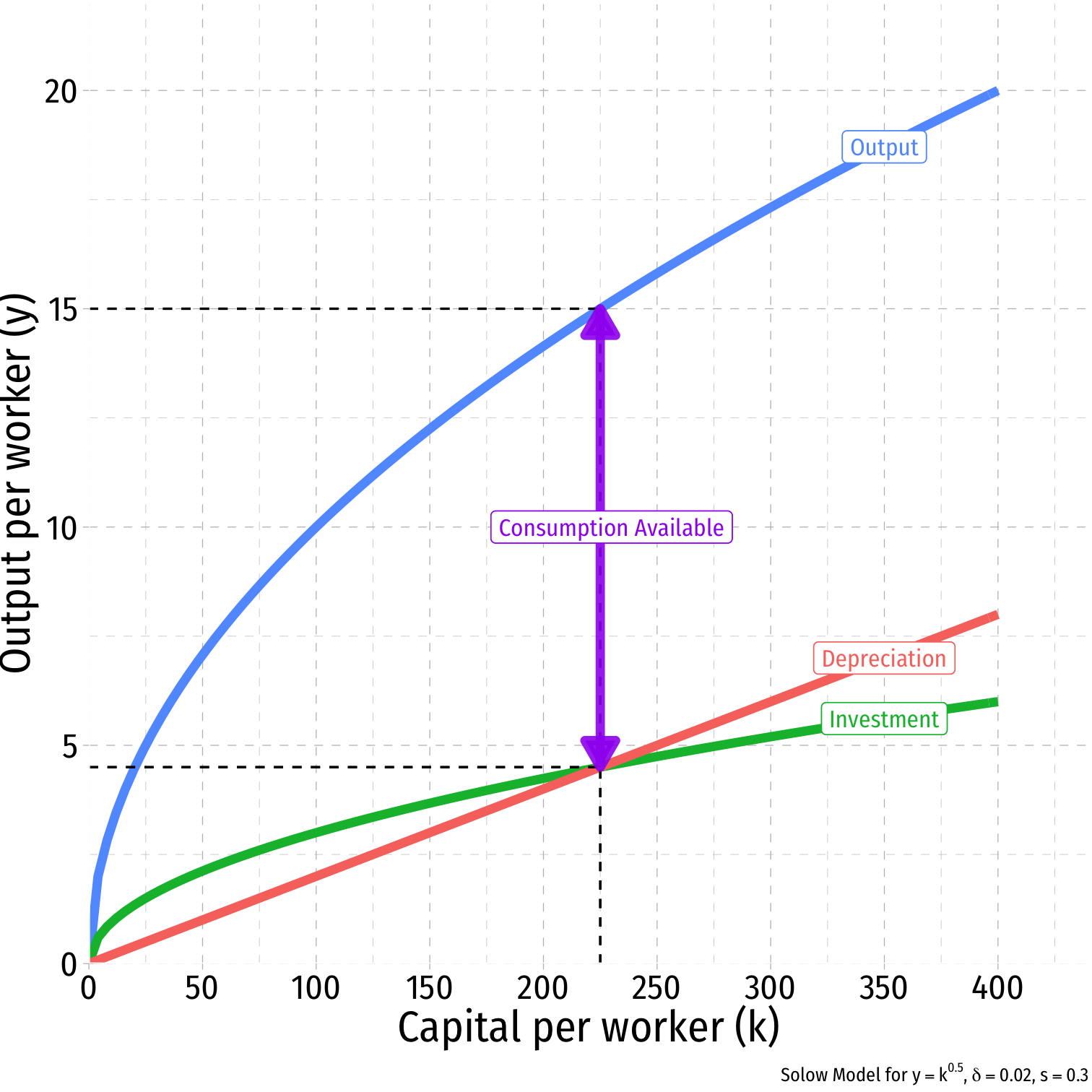
Graphically: Capital & the Steady State

  • Whenever Investment = Depreciation

    • Capital stock reaches a steady state, gK=0
    • Adding exactly as much new capital that is lost to depreciation
    • No movement on graph

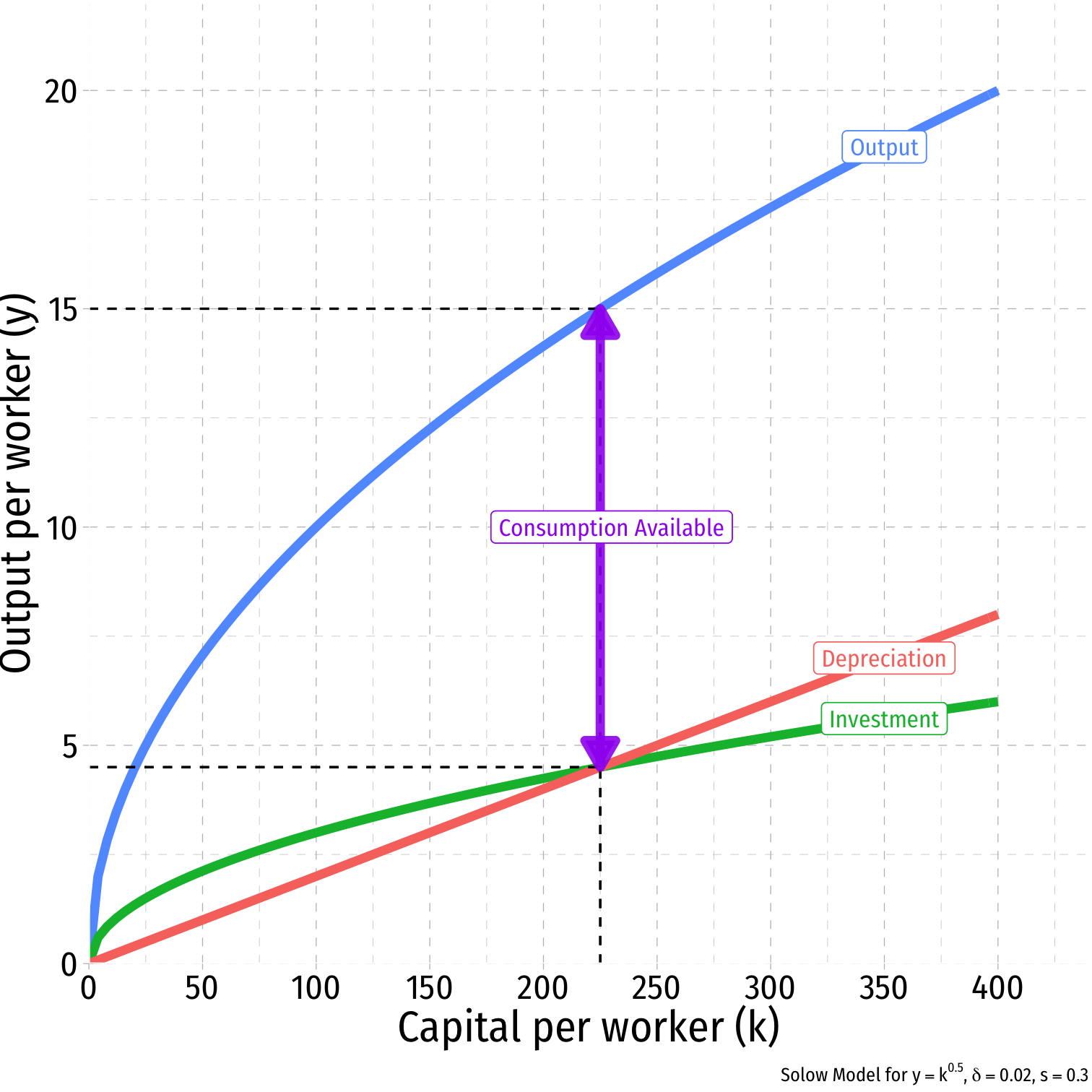
  • Steady State level of capital: k∗t:sf(kt)=δkt,gk=0

  • Steady State level of output

    • Amount available for consumption, c∗t=y∗t−i∗t

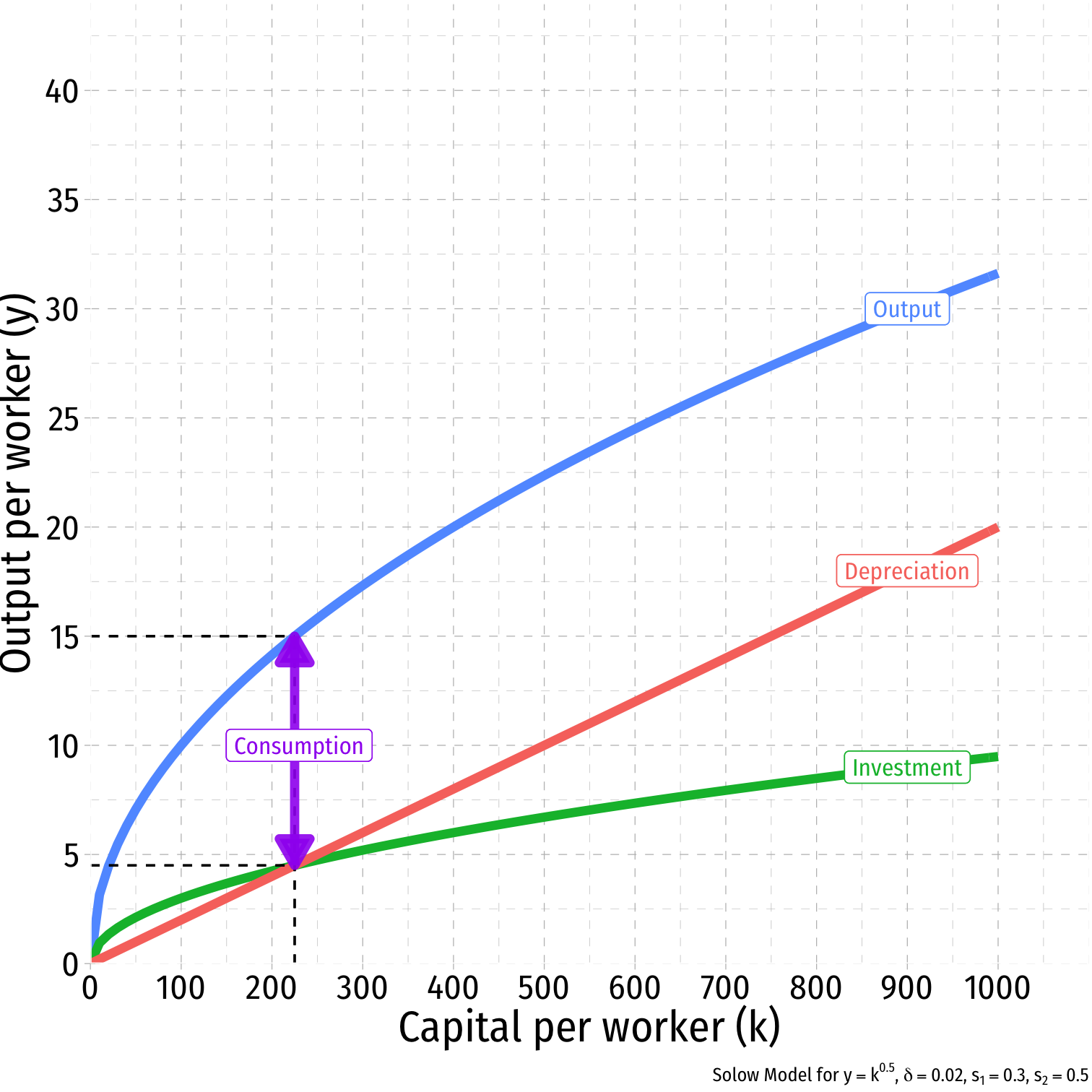
Comparative Statics: A Change in Savings I

  • What if consumers decide to save more?
    • s1=0.30
    • s2=0.50

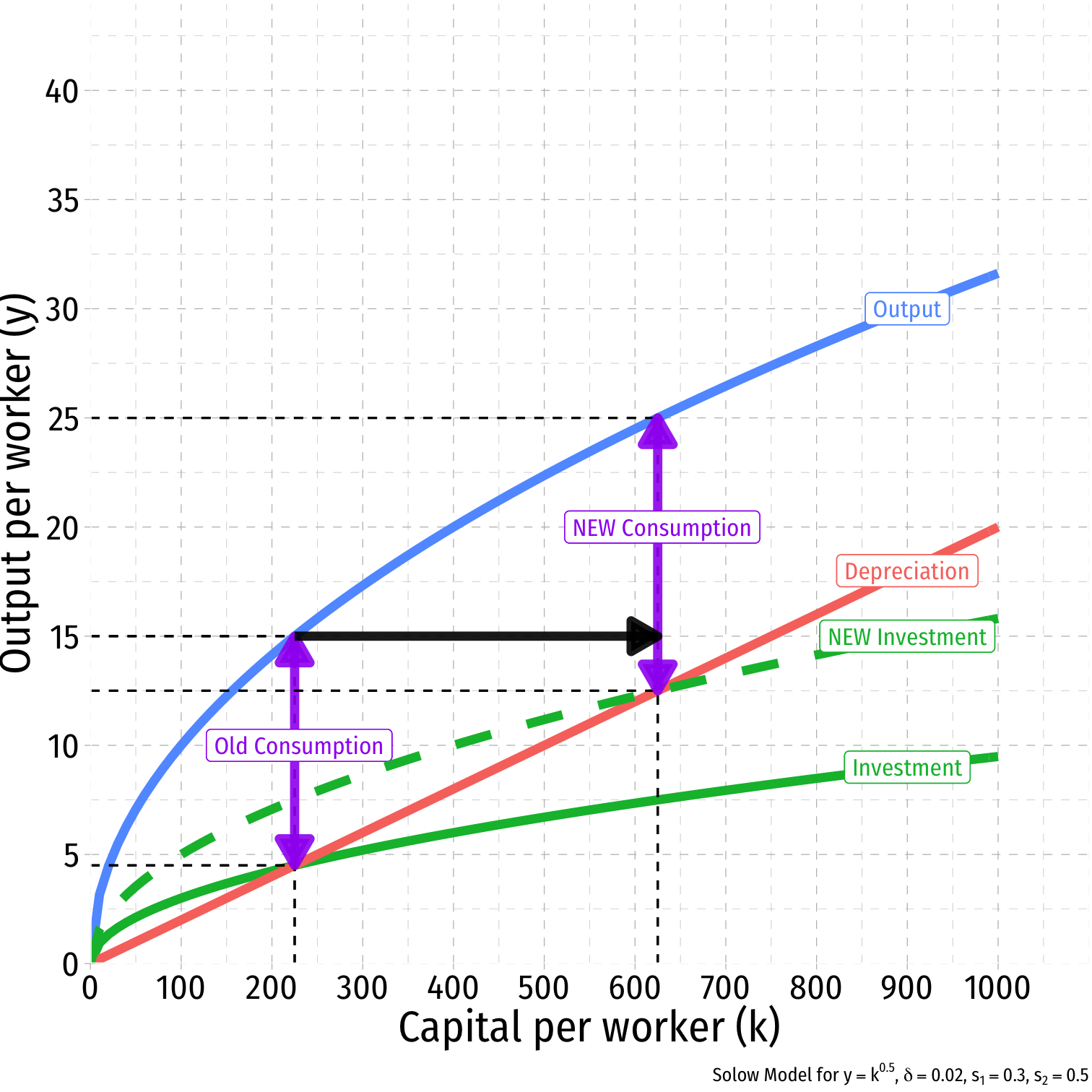
Comparative Statics: A Change in Savings II

  • What if consumers decide to save more?

    • s1=0.30
    • s2=0.50

  • Investment it increases

  • Steady state level of capital k∗t increases

  • Steady state output increases y∗t

  • Steady state amount of consumption

    • Decreases at first from more savings
    • Increases from more output produced

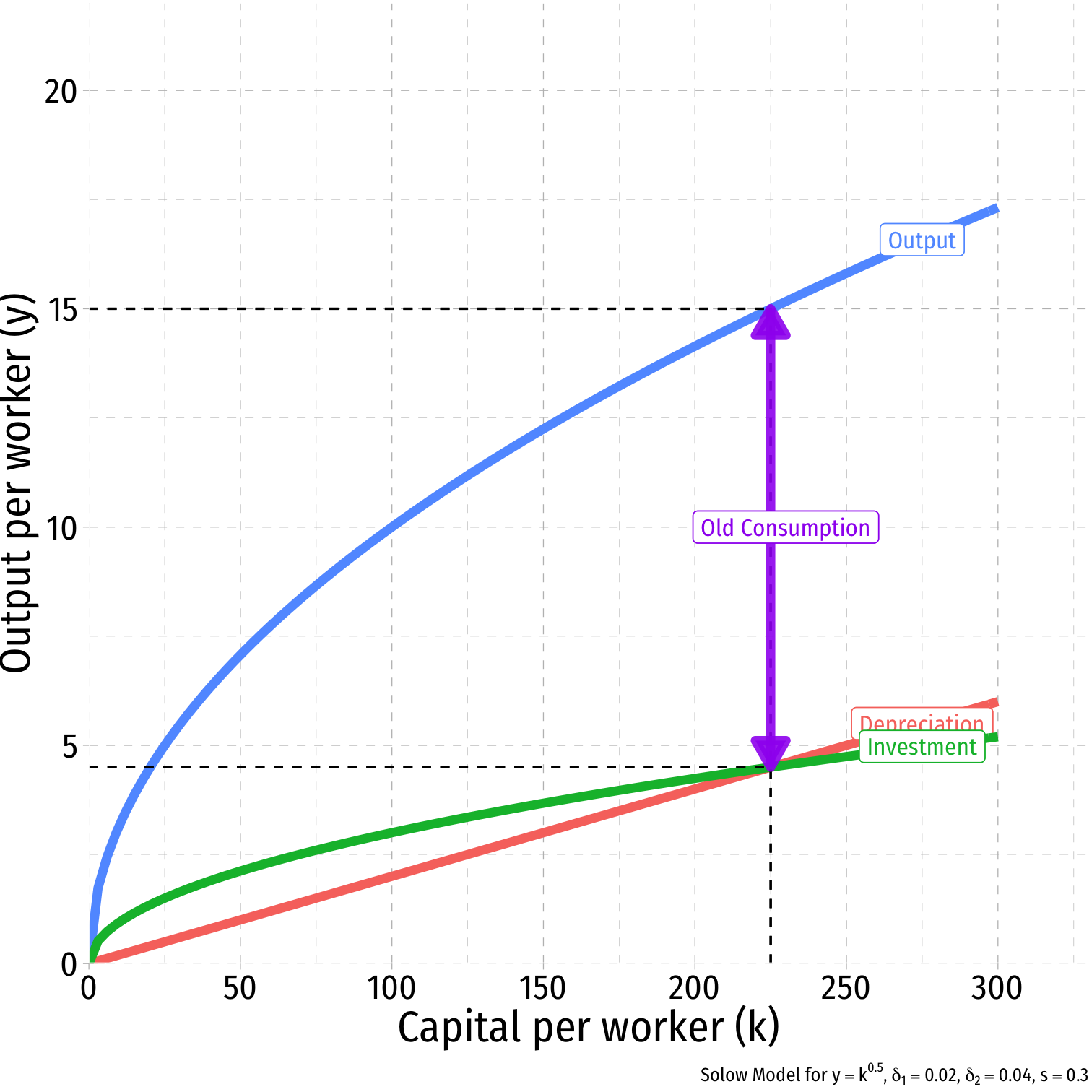
Comparative Statics: A Change in Depreciation I

  • What if depreciation costs increase?
    • δ1=0.02
    • δ2=0.04

Comparative Statics: A Change in Depreciation II

  • What if depreciation costs increase?

    • δ1=0.02
    • δ2=0.04

  • Investment it decreases

  • Steady state level of capital k∗t decreases

  • Steady state output decreases y∗t

  • Steady state amount of consumption c∗t decreases

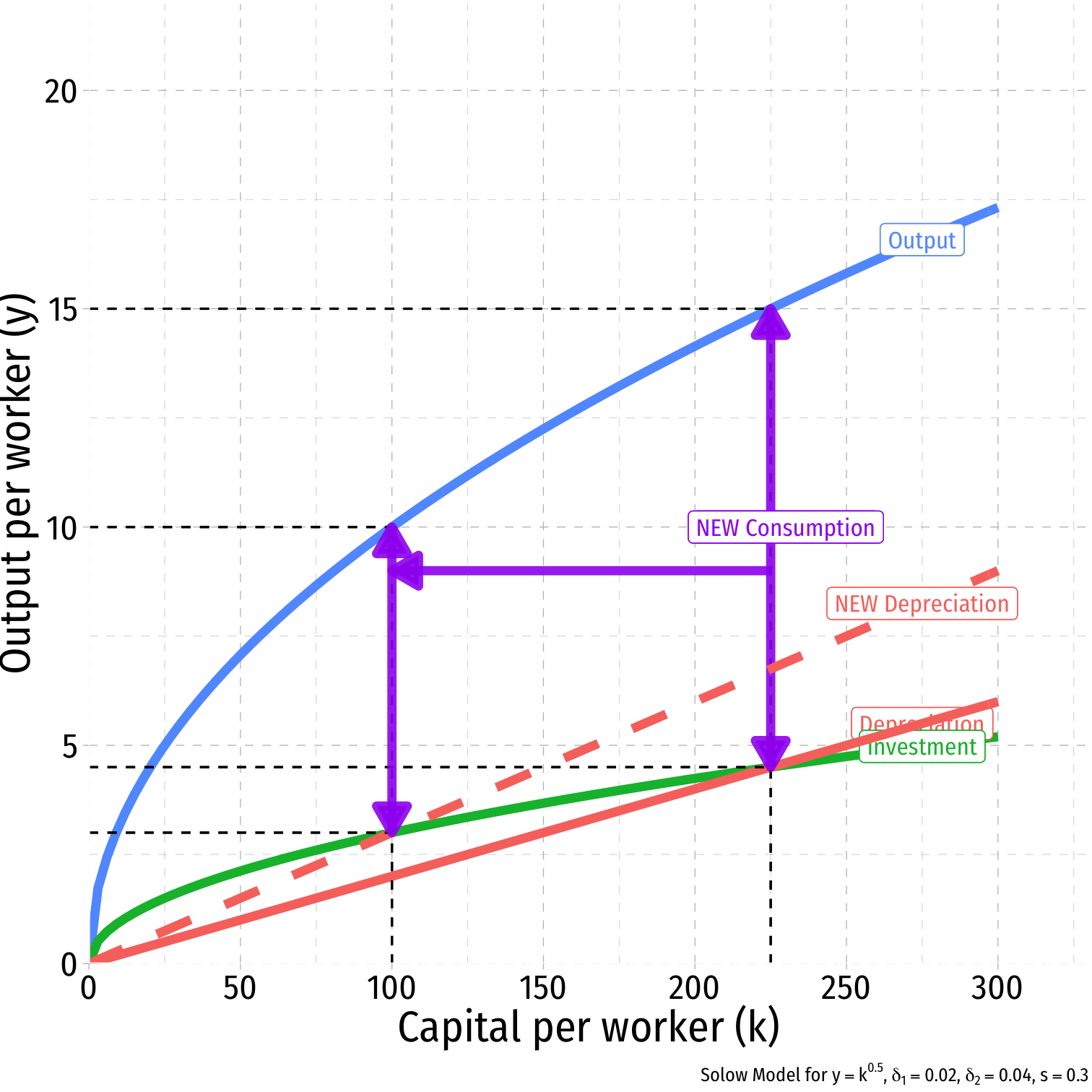
The Golden Rule Level of k I

  • Different values of s lead to different steady state levels of k∗, so which is best?

  • The best steady state is one where there is the highest possible consumption per person c∗=(1−s)f(k∗)

  • Increase in s

    • Reduces consumption's share of income (1−s)
    • Results in higher k∗ and higher y∗

  • Find the value of s (and k∗) that maximizes c∗

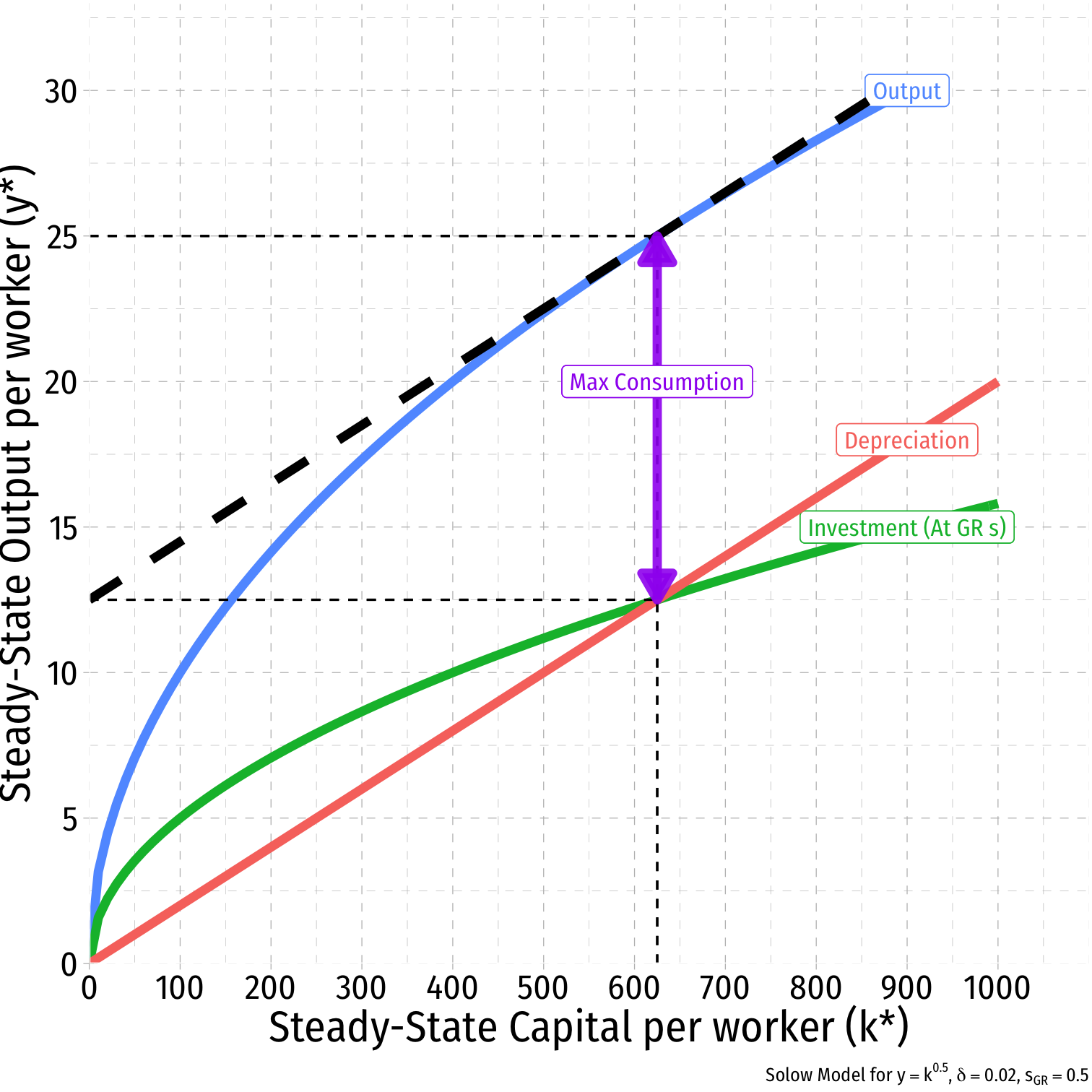
The Golden Rule Level of k II

maxc∗c∗=f(k∗)⏟y∗−δk∗⏟=i∗ in SS

dc∗dk∗=df(k∗)dk∗−dδk∗dk∗0=MPK−δMPK=δ

  • Golden Rule level of k∗GR where slope of depreciation line = slope of production function, f(k∗)

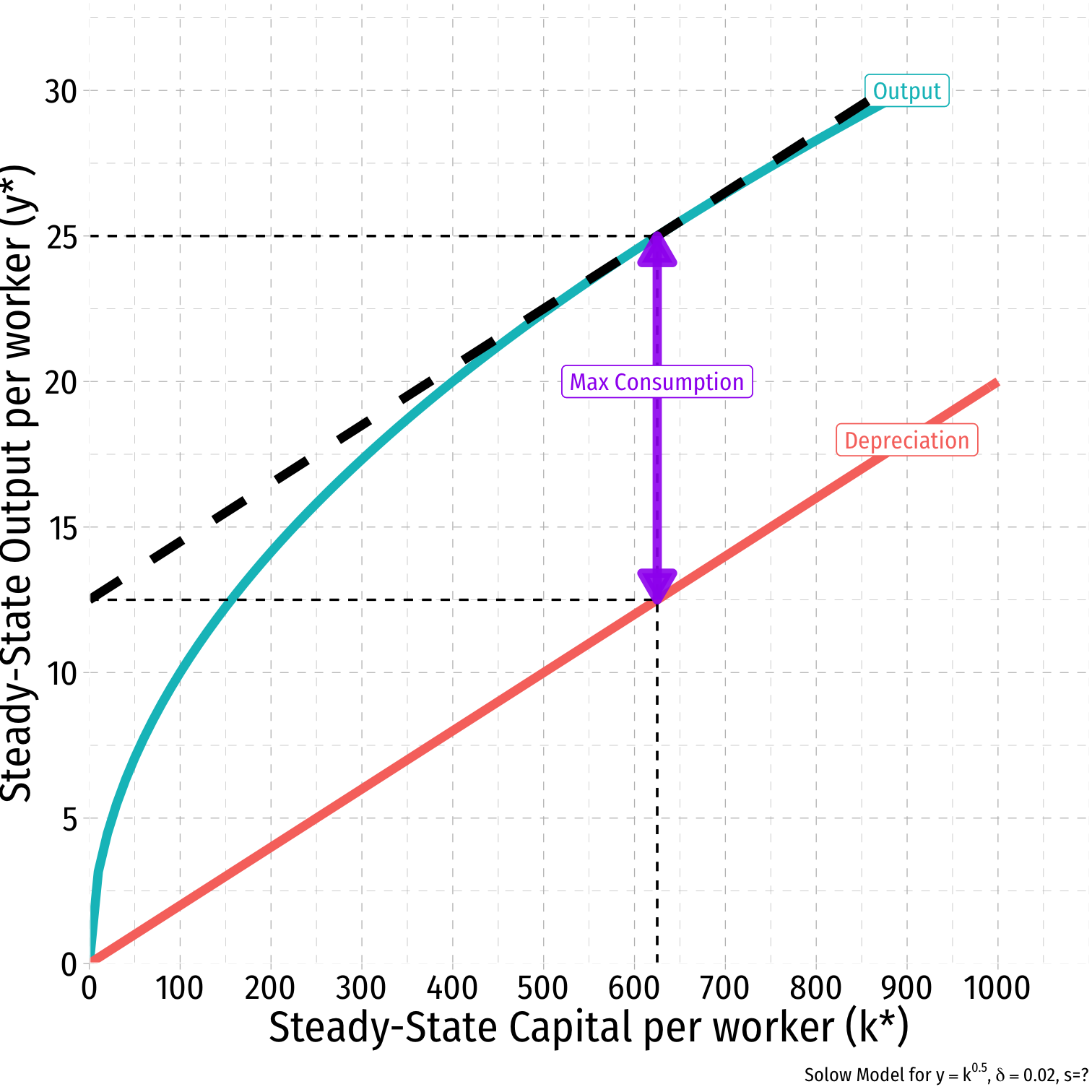
The Golden Rule Level of k III

  • Golden Rule level of k∗GR where slope of depreciation line = slope of production function, f(k∗)

  • Golden Rule level of k∗GR=δk∗GRy∗GR

    • In this example, sGR=0.02(625)25=0.50

  • Optimal level of savings is 0.50 or 50%!

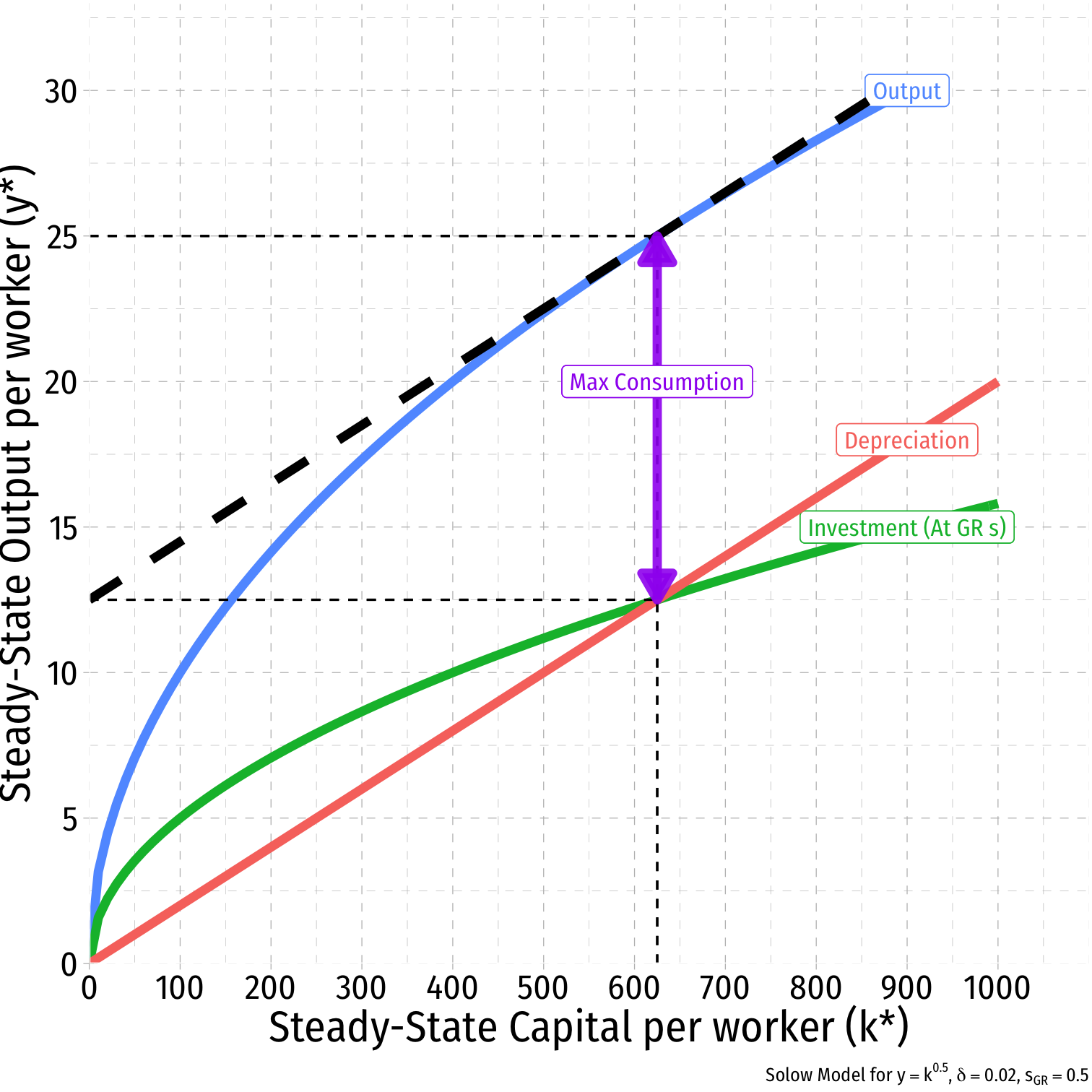
The Golden Rule Level of k IV

  • Policy implications: policymakers can choose s to maximize c∗i at k∗GR

  • Change taxes or government spending

Main Properties of the Solow Model

  1. There exists a unique steady state capital to labor ratio, k∗

    • Where investment = depreciation
    • sf(k)=δ(k)

  2. Higher savings rate s implies a higher steady state value of k∗

  3. An economy converges over time to the steady state level of k∗

The "Simple" Solow Model and Kaldor's Facts

  1. In steady state, gy=0 and gk=0: output and capital (per worker) do now grow!

  2. The only explanation that fits with Kaldor's facts (1-2) is that all countries must be BELOW their steady states

  3. Growth would have to be slowing down over time

  • These are motivations for the "full Solow" model

The “Full” Solow Model

The “Full” Solow Model I

  • Add two new "laws of motion" beyond just capital:

  • Population grows at constant rate n over time

    • Lt+1=Lt(1+n)
    • gL=n

  • Technology grows at constant rate g over time

    • At+1=At(1+g)
    • gA=g (g: growth rate of technology)

  • Redefine k≡KtAtLt as capital per effective worker

    • Labor augmented by technology, hence At×Lt
    • At+1Lt+1=AtLt(1+n)(1+g)

The “Full” Solow Model II

  • Long story short, our new takeaway implications:

  1. Δk=sf(kt)−(δ+n+g)kt

    • Capital per effective worker is equal to investment (first term) minus break-even investment

  2. Break even investment: (δ+n+g)k

    • Amount of investment necessary to keep k constant, consists of:
      • δk: to replace capital depreciation
      • nk: to provide capital to new workers
      • gk: to provide capital for new "effective workers" created by technology

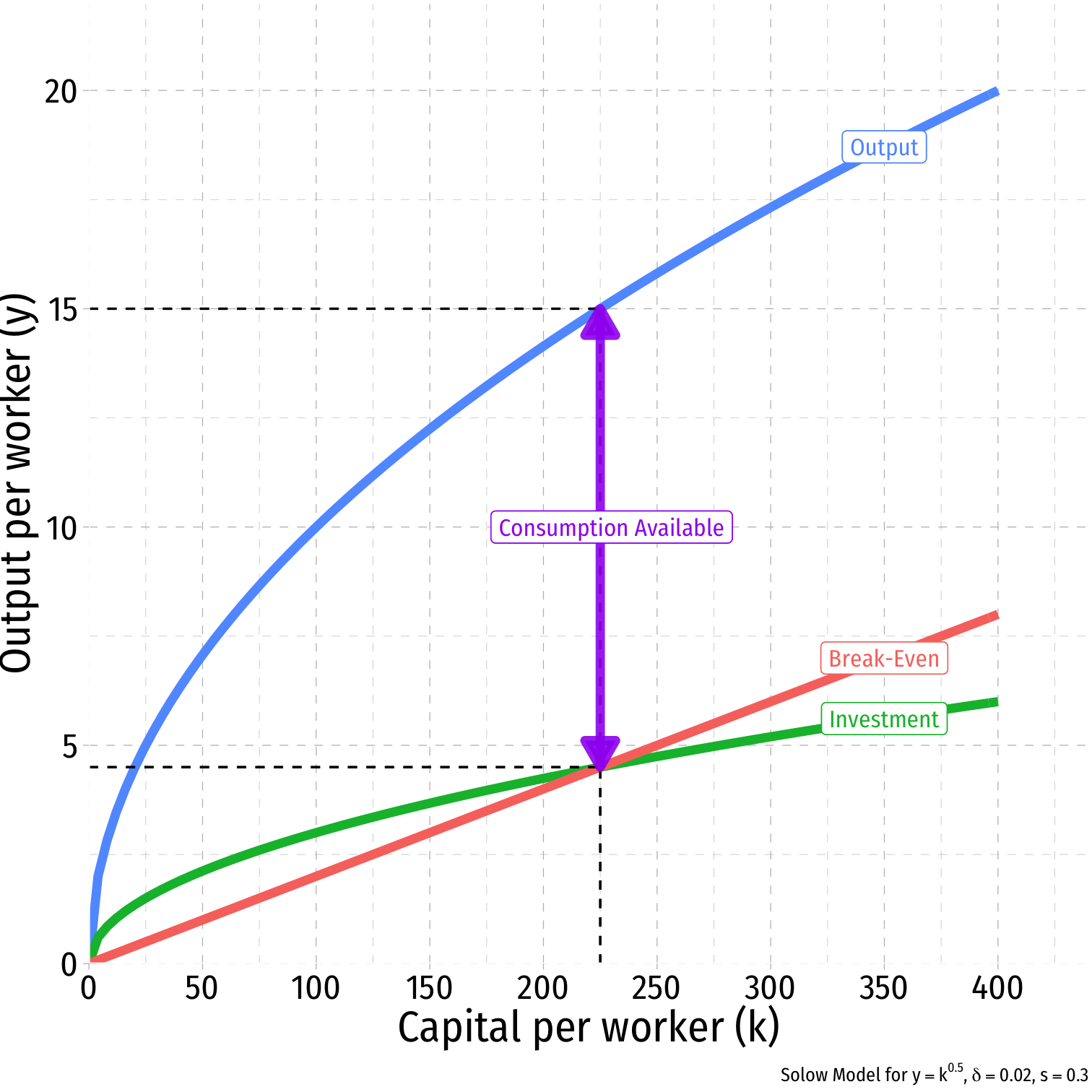
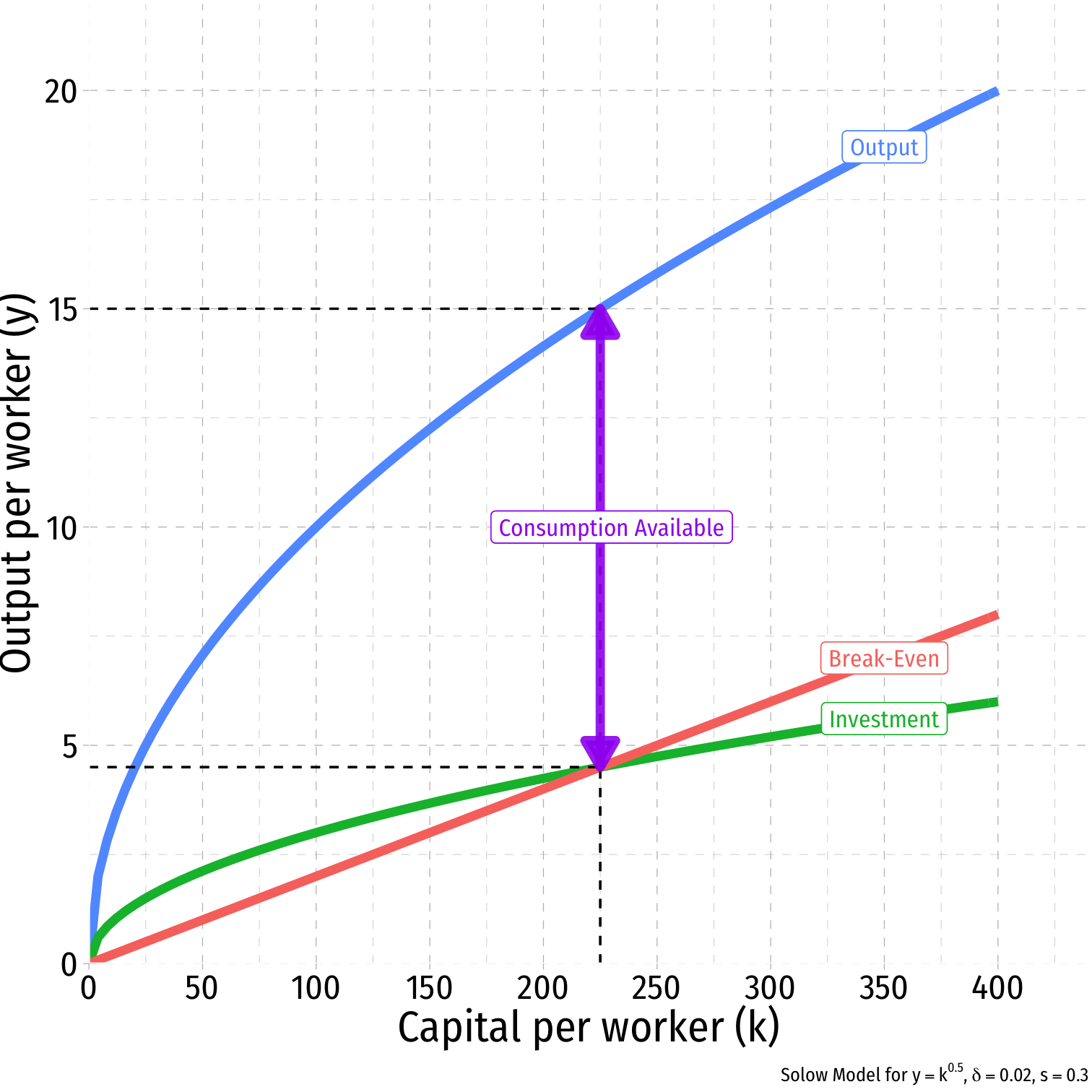
The “Full” Solow Model: Graphically I

  • Whenever Investment = Break-even Investment

    • Capital stock reaches a steady state, gK=0
    • Adding exactly as much new capital that is needed to break-even
    • No movement on graph

  • Steady State level of capital: k∗t:sf(kt)=(δ+n+g)kt,gk=0

  • Steady State level of output

    • Amount available for consumption, c∗t=y∗t−i∗t

The “Full” Solow Model: Graphically II

  • Growth rates in the steady state:
Variable Symbol Growth Rate
Capital per effective worker k=KAL 0
Output per effective worker y=YAL 0
Output per worker YL=Ay g
TFP A g
Labor (population) L n
Total Capital K=ALk n+g
Total Output Y=yAL n+g
  • Interesting: growth rate of output per worker grows solely from rate of TFP progress (g)!

The “Full” Solow Model and Kaldor's Facts

  1. Output per worker grows at rate g (Kaldor's Fact 1)

  2. Capital per worker grows at rate g (Kaldor's Fact 2)

  3. Capital and output grow at the same rate over time (Kaldor's Fact 3)

  4. Capital and labor's share of output (α and 1−α, respectively) do not change over time (Kaldor's Fact 4)

  5. The return to capital is constant (it can be shown to be r=α(k∗)α−1)

  • What about Kaldor's Fact 6: levels of output per worker vary widely across countries?

Cross-Country Comparisons

Solow Model Cross-Country Comparisons: Convergence

  • All else equal, poor countries (low YL and KL) should grow faster than rich ones (high YL and KL)

  • Income gap between wealthy and poor countries should cause living standards to converge over time

Convergence: Technical

  • Near the balanced growth path k→(k∗) at a speed proportional to its distance from k∗:

k(t)≈k∗+e−[1−αk∗](n+g+δ)t(k0−k∗)

  • In other words - the further away from (closer to) (k^*) your country is, the faster (slower) you should grow

Convergence? I

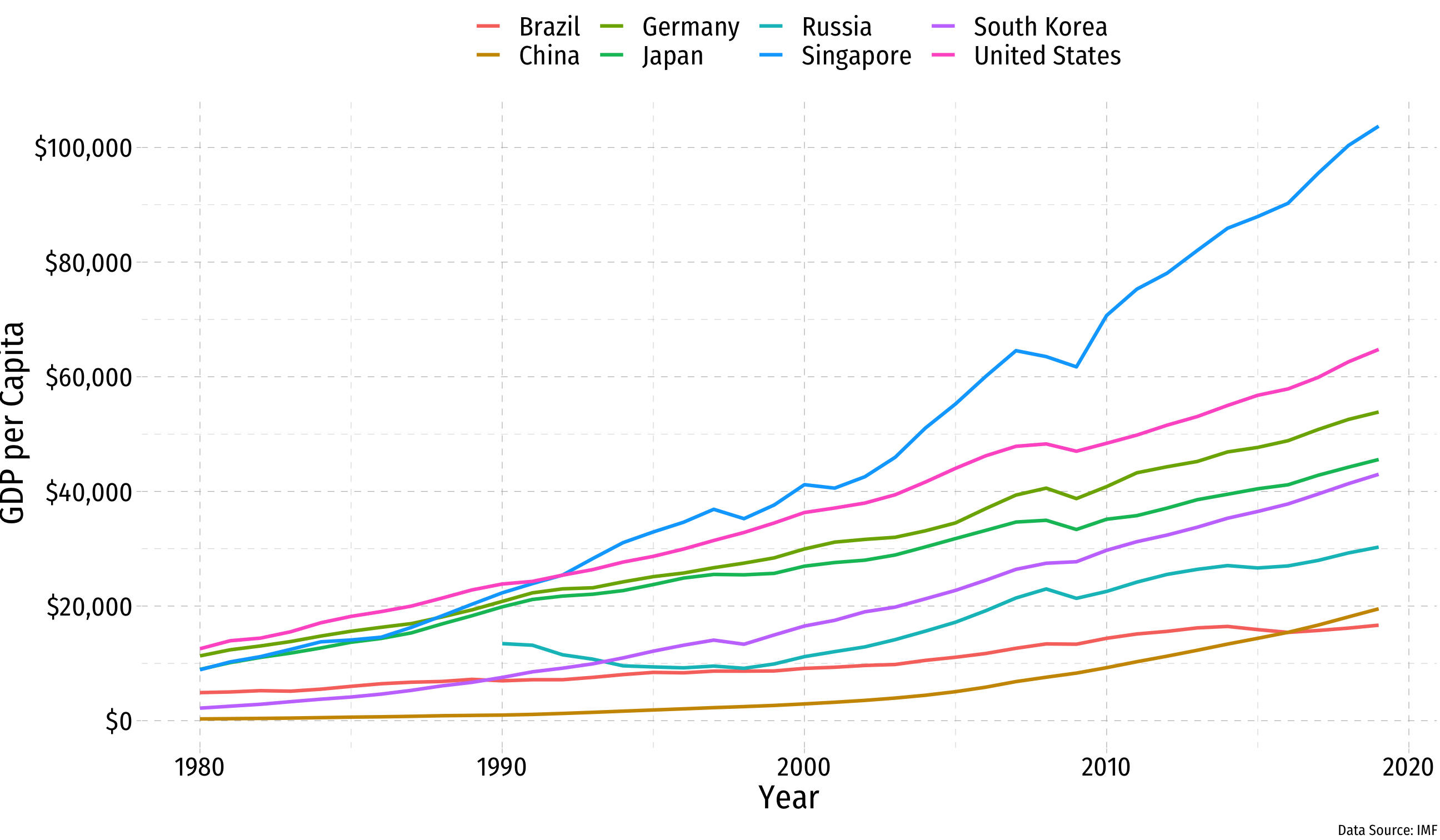
Convergence? II

James Bessen

1958-

"By the early twentieth century, British textile equipment manufacturers were shipping power looms and other textile equipment around the globe. Mills in India, China, and elsewhere not only used the same equipment as British mills, but they were often run by experienced British managers aided by British master weavers and spinners and engineers. Nevertheless, their output per worker was far less than that of the English or American mills because their workers -- using the exact same machines -- lacked the same knowledge and skills. Western weavers were 6.5 times more productive. The English and American cotton textile industries held a sustained economic advantage for decades, despite paying much higher wages," (pp.18-19).

Bessen, James, 2015, Learning by Doing: The Real Connection between Innovation, Wages, and Wealth, New Haven, CT: Yale University Press

Convergence? III

James Bessen

1958-

"[T]he technical knowledge needed to install, manage, and operate this technology, along with the necessary institutiosn and organizations to allow large numbers of workers to acquire this knowledge, did not appear in these countries for many decades. Cotton textile workers in China, India, and Japan in 1910 had the same machines as those in England, but their productivity was far less than that of the English or American workers because they lacked the same knowledge and skills. Even when English managers ran mills in India and China, productivity tended to be low because the English managers had to adapt their knowledge to a different environment and culture.," (p.98).

Bessen, James, 2015, Learning by Doing: The Real Connection between Innovation, Wages, and Wealth, New Haven, CT: Yale University Press

Convergence? IV

  • All else is not equal!

  • Solow model predicts conditional convergence: countries converge to their own steady states determined by saving, population growth, and education (s,n,g)

  • IF countries had similar institutions, then they should converge

From MR University

Conditional Convergence

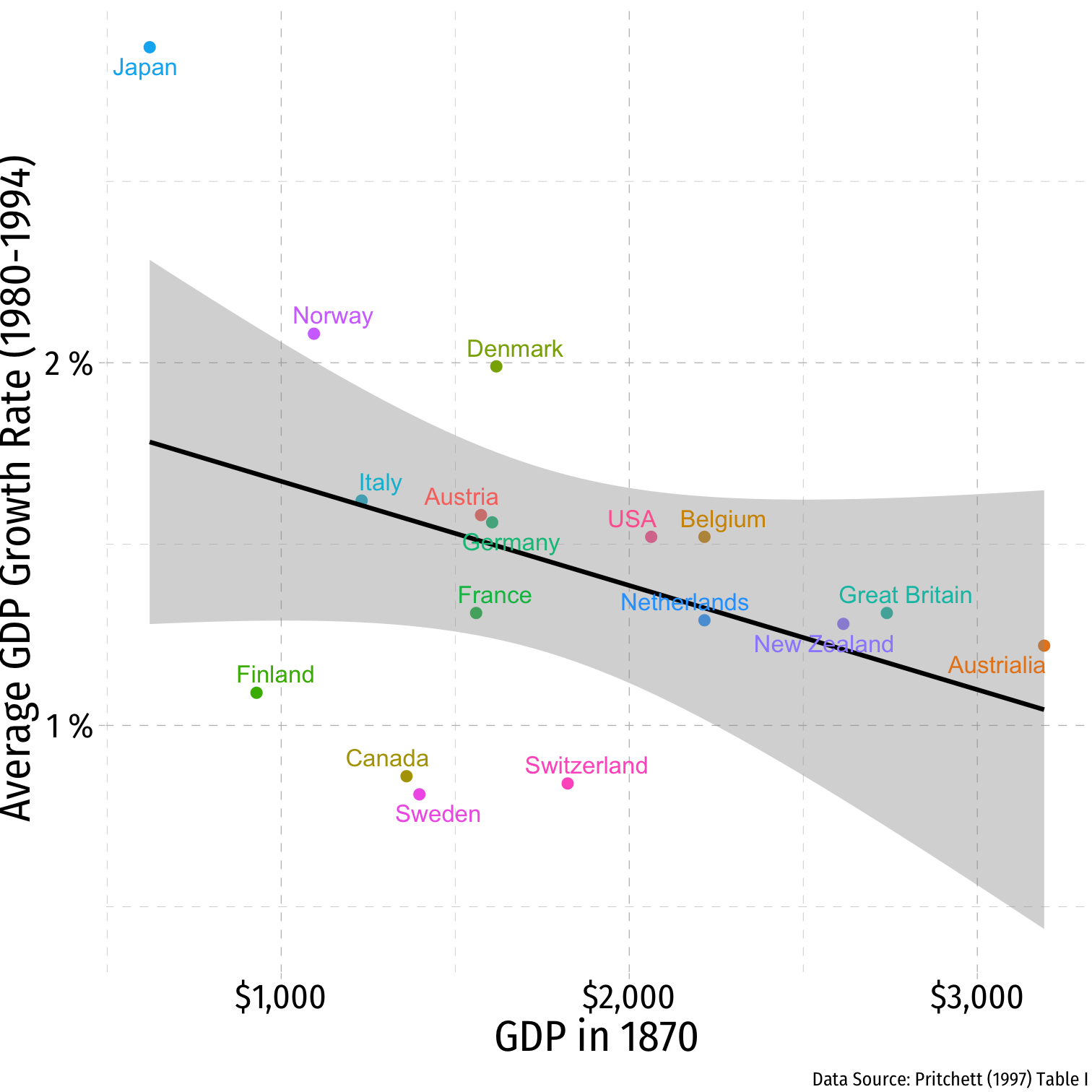
Pritchett, Lant, 1997, "Divergence, Big Time," Journal of Economic Perspectives 11(3): 3-17

Divergence, Big Time

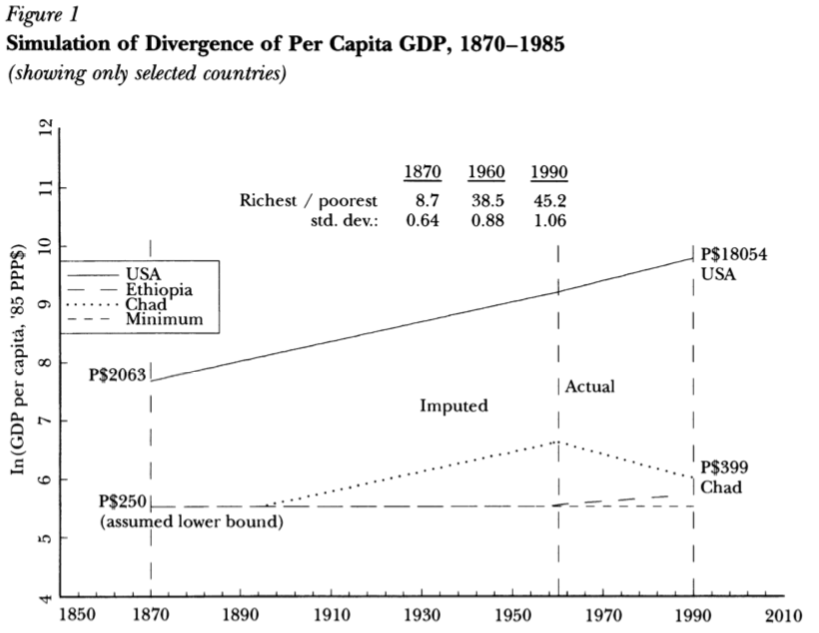
"[F]rom 1870 to 1990 the ratio of per capita incomes between the richest and the poorest countries increased by roughly a factor of five and that the difference in income between the richest country and all others has increased by an order of magnitude."

Pritchett, Lant, 1997, "Divergence, Big Time," Journal of Economic Perspectives 11(3): 3-17

And Now Convergence, Big Time?

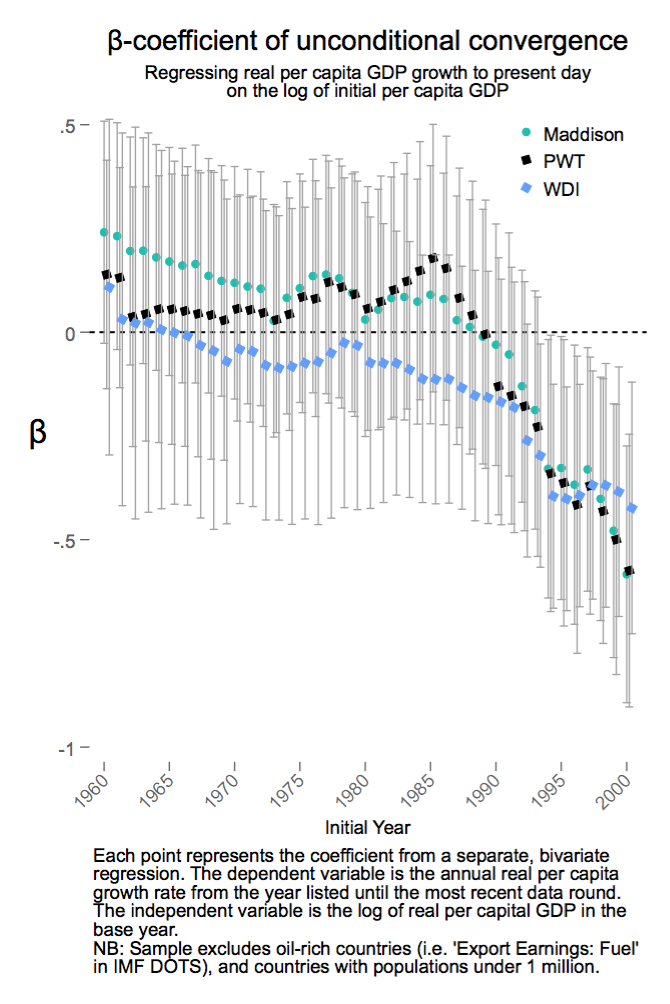
"While unconditional convergence was singularly absent in the past, there has been unconditional convergence, beginning (weakly) around 1990 and emphatically for the last two decades."

Johnson, Paul and Chris Papageorgiou, 2018, "What Remains of Cross-Country Convergence?" Journal of Economic Literature, forthcoming

Convergence

  • Consider two types of economic growth

  • "Cutting-edge Growth"

    • tends to be much slower
    • has to push out the PPF with new innovation and progress

  • "Catching-up Growth"

    • tends to be much faster
    • can mimic and import existing innovation from other countries

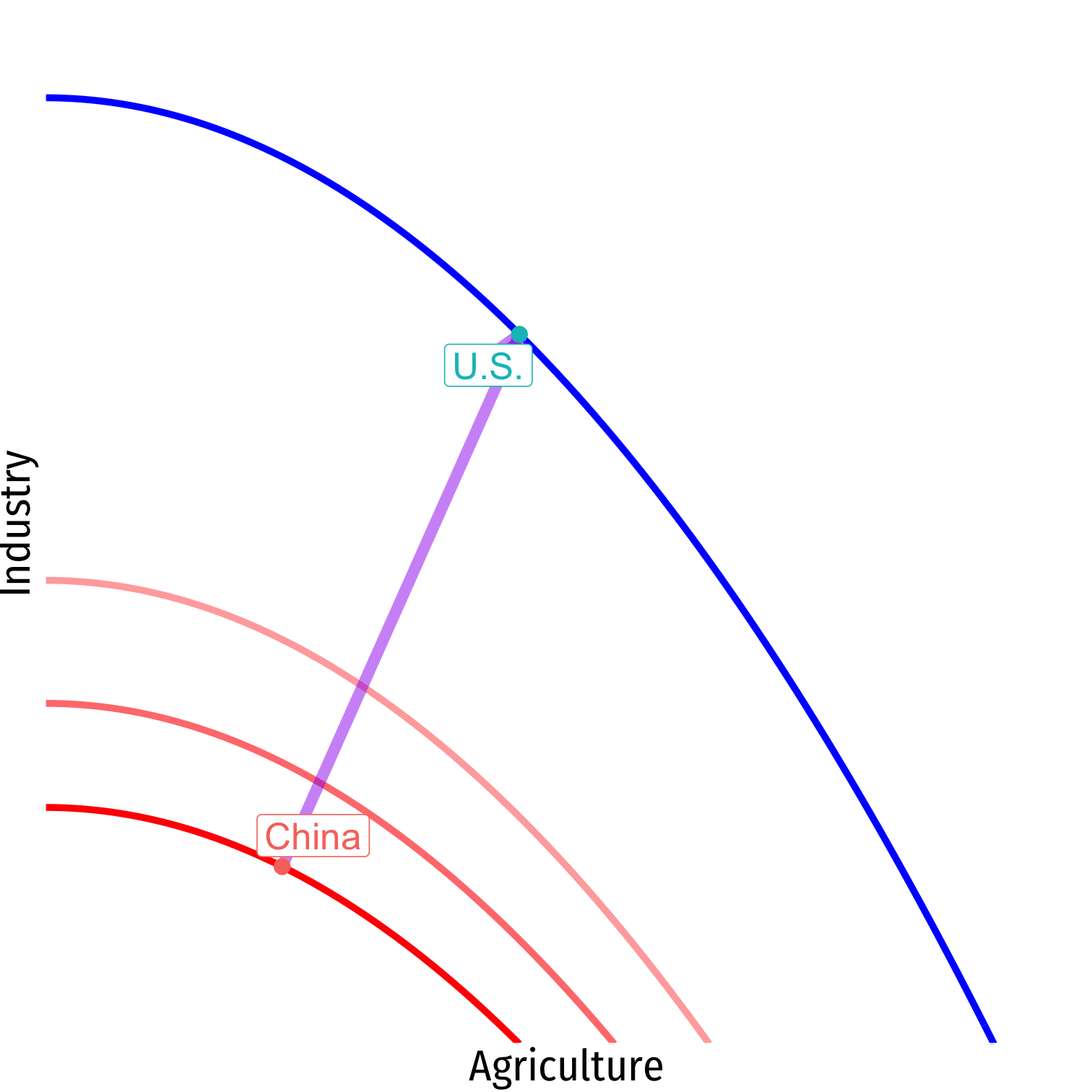
Growth on the Frontier is Hard I

Source: The Atlantic (Nov 16, 2018)

Source: Freakonomics (Nov 29, 2017)

Growth on the Frontier is Hard II

Growth Accounting

The Solow Model: Growth Accounting I

gY=FAAY∗gA+αgK+(1−α)gL

  • Output growth gY can be explained as the growth of "technology" gA and the growth of factors (αgK+(1−α)gL)1

1 All g's stand for growth rates, or percentage change, of the relevant variable (Y,A,K,L). See the class notes page for a derivation of Growth Accounting based on Solow (1957)

The Solow Model: Growth Accounting I

gY=FAAY∗gA+αgK+(1−α)gL

  • Output growth gY can be explained as the growth of "technology" gA and the growth of factors (αgK+(1−α)gL)1

1 All g's stand for growth rates, or percentage change, of the relevant variable (Y,A,K,L). See the class notes page for a derivation of Growth Accounting based on Solow (1957)

  • Used to determine how much of total output can be explained by growth in factors and "everything else," known as the Solow Residual - often interpreted as "technology"

The Solow Model: Growth Accounting I

gY=FAAY∗gA+αgK+(1−α)gL

  • Output growth gY can be explained as the growth of "technology" gA and the growth of factors (αgK+(1−α)gL)1

1 All g's stand for growth rates, or percentage change, of the relevant variable (Y,A,K,L). See the class notes page for a derivation of Growth Accounting based on Solow (1957)

  • Used to determine how much of total output can be explained by growth in factors and "everything else," known as the Solow Residual - often interpreted as "technology"
  • We can directly measure (roughly) Y,L,K and α, but not FAAY, the Solow residual
    • Measure it as Solow Residual=gY−αgK−(1−α)gL

Solow, Robert, 1957, "Technical Change and the Aggregate Production Function," The Review of Economics and Statistics 39(3): 312-320

The Solow Model: Growth Accounting II

Robert Solow

(1924-)

Economics Nobel 1987

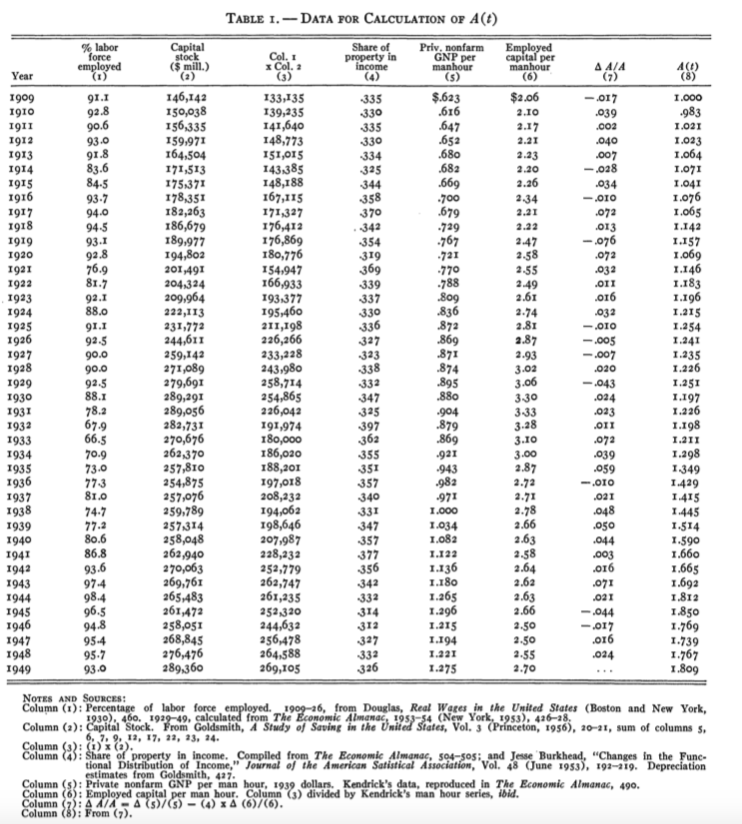
Solow, Robert, 1957, "Technical Change and the Aggregate Production Function," The Review of Economics and Statistics 39(3): 312-320

The Solow Model: Growth Accounting III

Robert Solow

(1924-)

Economics Nobel 1987

Solow's findings for 1909-1949 in the United States:

  1. Output per worker grew by about 100%
  2. Capital-to-labor ratio grew by about 30% ("capital-deepening")
  3. Technology grew by about 87.5%
    • i.e. 87.5% of the growth in output per worker came from Technology; 12.5% from increases in capital per worker
  4. Measure of Technology fell in a number of recession/depression years and rose during expansions -- technology is "pro-cyclical"
  5. Aggregate production function displays a positive and diminishing marginal product of capital

Solow, Robert, 1957, "Technical Change and the Aggregate Production Function," The Review of Economics and Statistics 39(3): 312-320

The Solow Model: Let's Try Some Growth Accounting

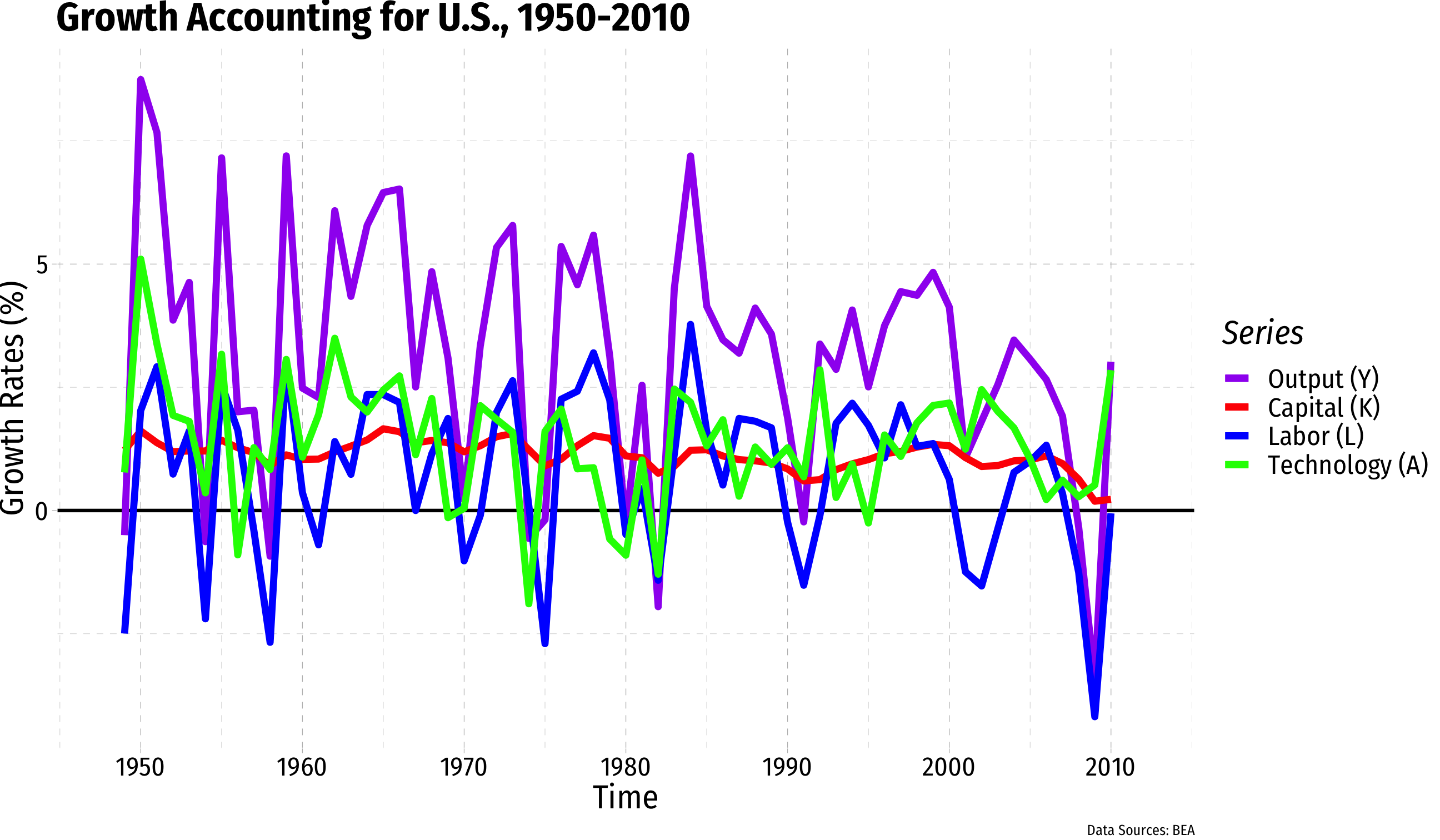
The Solow Model: Let's Try Some Growth Accounting

TFP in the U.S.

Source: Federal Reserve Bank of San Francisco

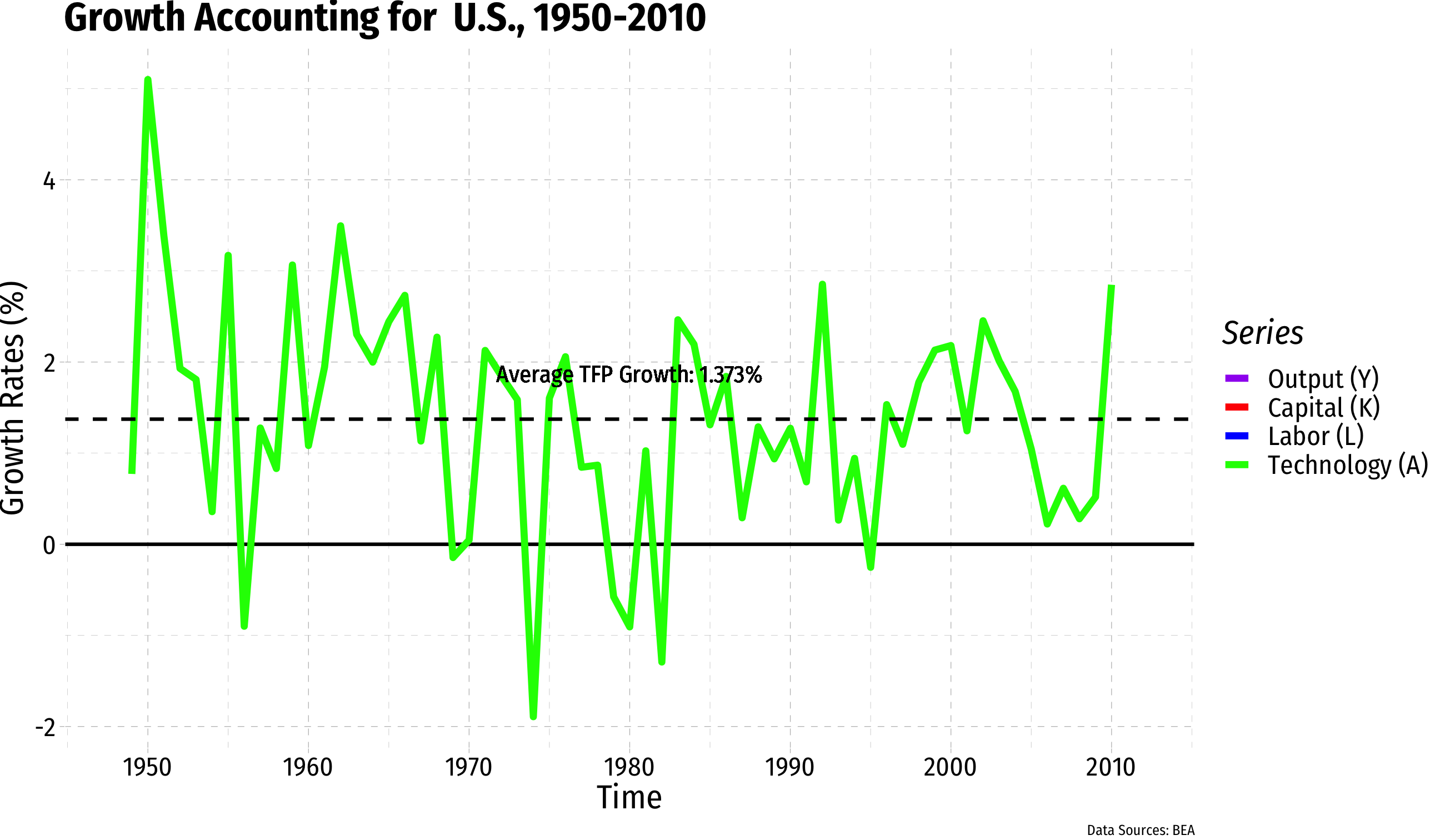
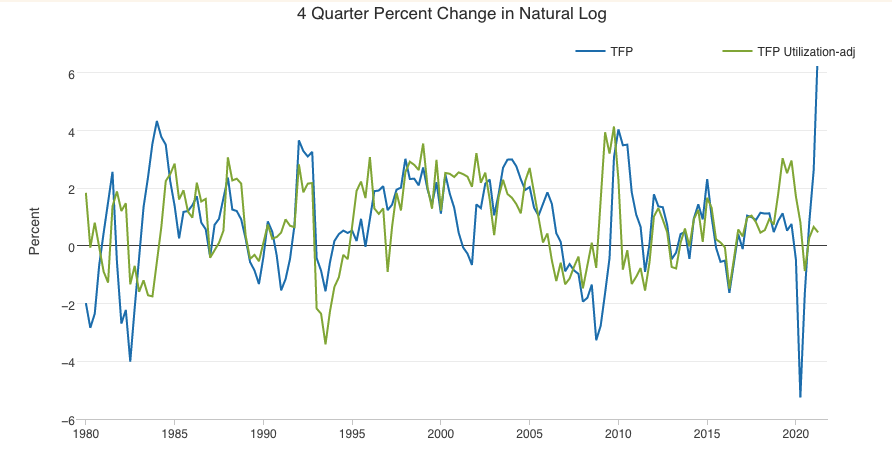
TFP in the U.S.: Not What It C/Should Be?

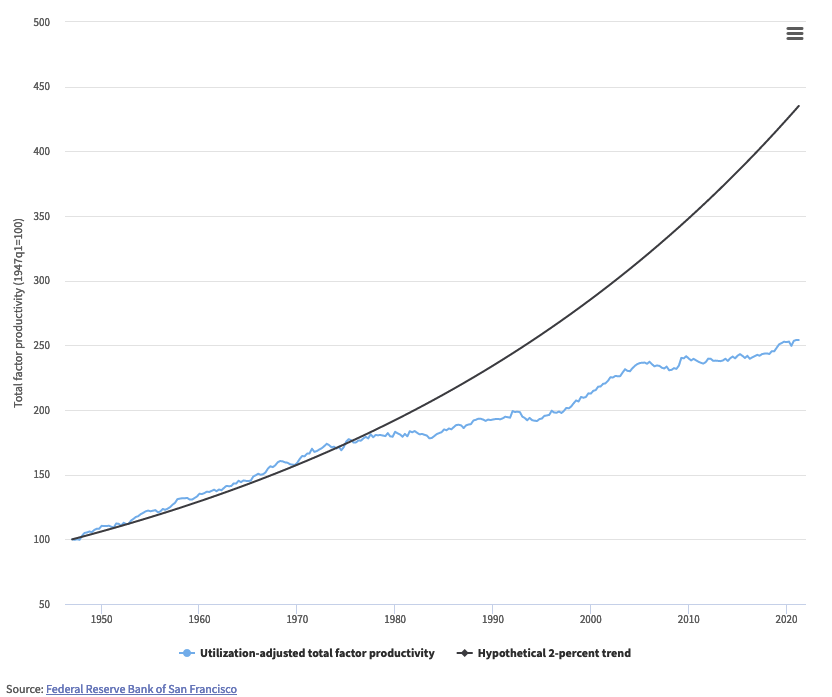
Sources: Eli Dourado; Federal Reserve Bank of San Francisco

Outline

Neoclassical Working Tools

The “Simple” Solow Model

The “Full” Solow Model

Cross-Country Comparisons

Growth Accounting

Paused

Help

Keyboard shortcuts

↑, ←, Pg Up, k Go to previous slide
↓, →, Pg Dn, Space, j Go to next slide
Home Go to first slide
End Go to last slide
Number + Return Go to specific slide
b / m / f Toggle blackout / mirrored / fullscreen mode
c Clone slideshow
p Toggle presenter mode
t Restart the presentation timer
?, h Toggle this help
oTile View: Overview of Slides
Esc Back to slideshow